Don't Count Now !!!
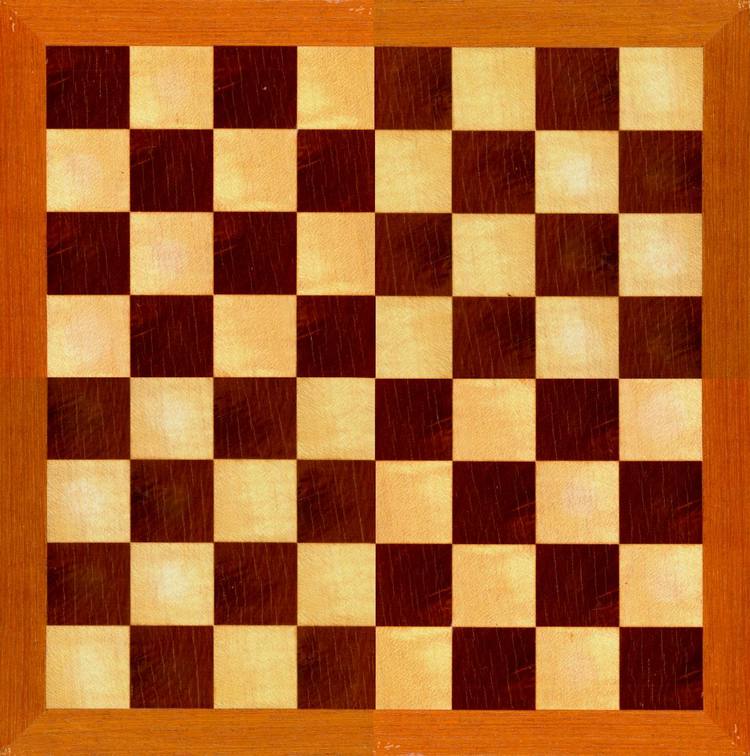 How many squares are there on a normal Chess Board ?
How many squares are there on a normal Chess Board ?
(Hint - Don't count just (8x8)=64 squares, there are other squares too !!!)
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
4 solutions
Yes Omkar !!
From where do you get all these stuff
No. of 1 × 1 squares = 6 4
No. of 2 × 2 squares = 4 9
No. of 3 × 3 squares = 3 6
No. of 4 × 4 squares = 2 5
No. of 5 × 5 squares = 1 6
No. of 6 × 6 squares = 9
No. of 7 × 7 squares = 4
No. of 8 × 8 squares = 1
So , T o t a l S q u a r e s = 6 4 + 4 9 + 3 6 + 2 5 + 1 6 + 9 + 4 + 1 = 2 0 4
The formula regarding the particular problem is n(n+1)(2n+1)/6.Therefore by substituting the values,we get the solution
There are 64, (1x1) squares, 49(2x2) squares, 36(3x3) squares, 25(4x4) squares, 16(5x5) squares, 9(6x6) squares, 4(7x7) squares, and, one BIG (8x8) square. So, the total no. of squares = 64+49+36+25+16+9+4+1=204. Hence, the correct answer is 204.
The number of squares on a n × n chess board is given by k = 1 ∑ n k 2