Dynamic Geometry: P8
 The diagram shows a yellow angle called
α
with
0
∘
≤
α
≤
9
0
∘
. We inscribe a red circle inside the angle so that its radius is always equal to
1
. When
tan
(
2
α
)
tan
(
α
)
=
1
5
1
9
3
2
0
0
, the distance between the angle's apex (pink) and the circle's center (cyan) can be expressed as
b
a
where
a
and
b
are coprime positive integers. Find
a
+
b
.
The diagram shows a yellow angle called
α
with
0
∘
≤
α
≤
9
0
∘
. We inscribe a red circle inside the angle so that its radius is always equal to
1
. When
tan
(
2
α
)
tan
(
α
)
=
1
5
1
9
3
2
0
0
, the distance between the angle's apex (pink) and the circle's center (cyan) can be expressed as
b
a
where
a
and
b
are coprime positive integers. Find
a
+
b
.
The answer is 50.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Thank you for posting !
Given that
tan 2 α tan α 1 5 1 9 tan α 1 5 1 9 ⋅ 1 − tan 2 2 α 2 tan 2 α 1 5 1 9 tan 2 2 α ⟹ tan 2 α ⟹ sin 2 α = 1 5 1 9 3 2 0 0 = 3 2 0 0 tan 2 α = 3 2 0 0 tan 2 α = 1 6 0 0 ( 1 − tan 2 2 α ) = 1 6 0 0 1 6 0 0 − 1 5 1 9 = 1 6 0 0 8 1 = 4 0 9 = 4 0 2 + 9 2 9 = 4 1 9
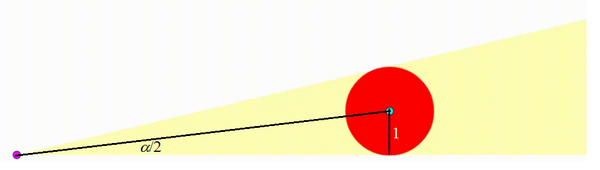
Note that the distance between the purple point and cyan point is sin 2 α 1 = 9 4 1 . Therefore a + b = 4 1 + 9 = 5 0 .
The double angle formula for tan is tan ( α ) = 1 − tan 2 ( α / 2 ) 2 tan ( α / 2 ) 1 − tan 2 ( α / 2 ) = 1 5 1 9 / 1 6 0 0 tan ( α / 2 ) = 9 / 4 0 y = x tan ( α / 2 ) = 1 d = x 2 + y 2 = 9 2 4 0 2 + 9 2 y = 9 4 1