Dynamic Geometry: P57
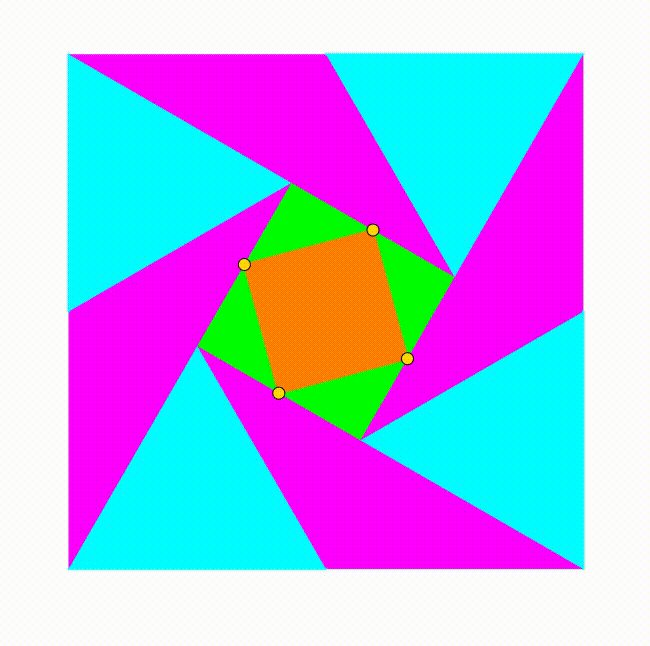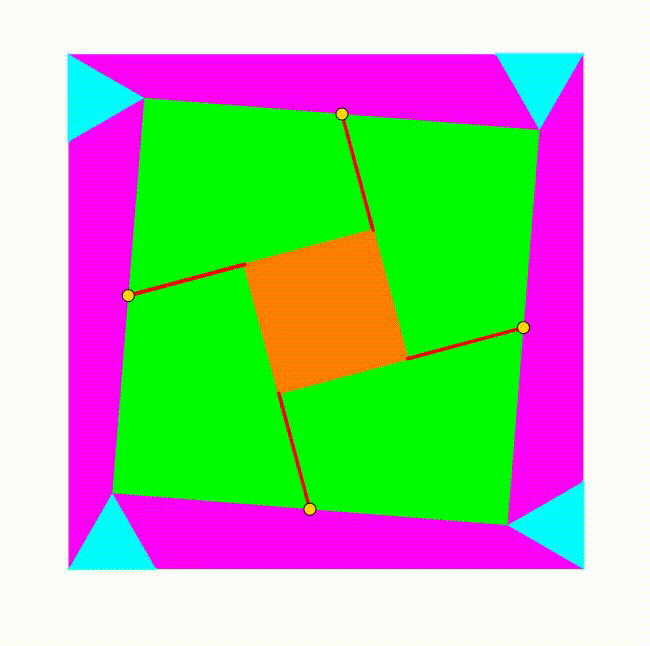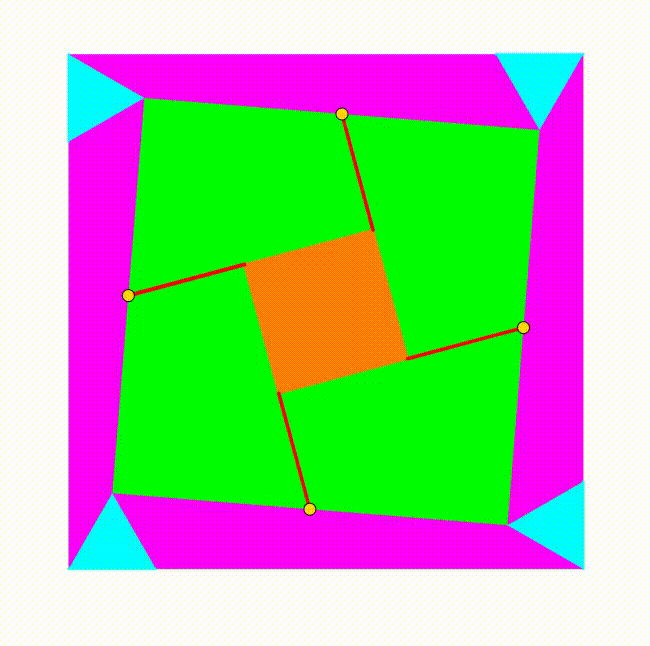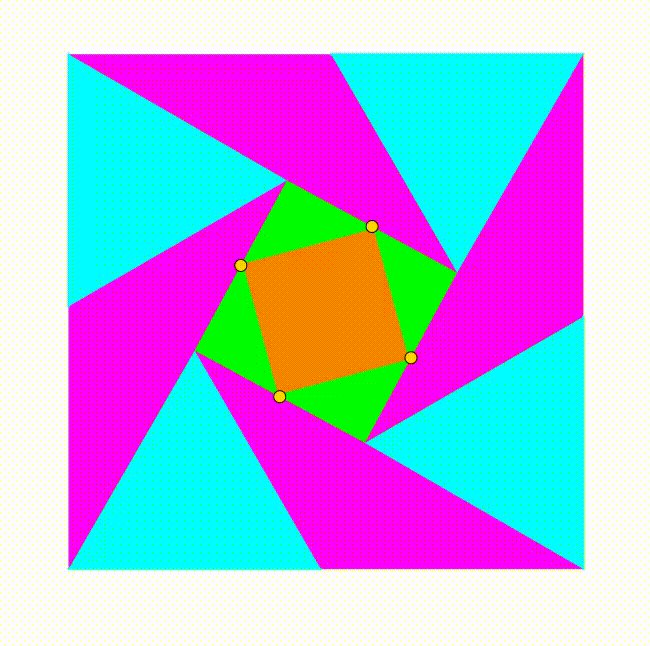
The diagram shows a unit square. Four equilateral triangles are growing and shrinking at the same rate so the quadrilateral drawn by joining their vertices is always a square (green). Each midpoint of the green square's sides traces a locus (red lines). The four intersection points between these red lines allows us to draw an orange square. The area of the orange square can be expressed as:
where , , and are positive integers. and are coprime and is square-free. Find .
The answer is 10.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let the center of the unit square be O ( 0 , 0 ) , the origin of the x y -plane, Let the vertices of top two variable equilateral triangle be A and B , and the midpoint of A B be P ( x , y ) . Let the side length of the two triangles be a , then A = ( 2 1 − 2 a , 2 1 − 2 3 a ) , B = ( − 2 1 + 2 3 , 2 1 − 2 a ) , and the coordinates of P ( x , y ) are:
⎩ ⎪ ⎪ ⎪ ⎪ ⎨ ⎪ ⎪ ⎪ ⎪ ⎧ x = 2 1 ( 2 1 − 2 a − 2 1 + 2 3 a ) = 4 3 − 1 a y = 2 1 ( 2 1 − 2 3 a + 2 1 − 2 a ) = 2 1 − 4 3 + 1 a ⟹ a ⟹ y = 3 − 1 4 x = 2 1 − ( 2 + 3 ) x
Therefore the locus of P is a fixed straight line. Let the side length of the orange square be b . Then b = 2 c cos θ , where c is the x -intercept of the locus and θ = tan − 1 2 + 3 1 . And x -intercept c is given by:
0 ⟹ c b = 2 1 − ( 2 + 3 ) c = 2 ( 2 + 3 ) 1 = 2 ( 2 + 3 ) 2 cos θ = 2 + 3 1 ⋅ 2 2 + 3 2 + 3 = 2 2 + 3 1
Then the area of the orange square b 2 = 4 ( 2 + 3 1 = 2 1 − 4 3 . Therefore p + q + m + n = 1 + 2 + 3 + 4 = 1 0 .