Does this relate to Heron?

$\begin{cases} a+b+c+d = 12 & ...(1) \\ a+b+c-d = 10 & ...(2) \\ a+b-c+d = -2 & ...(3) \\ a-b+c+d = 6 & ...(4) \\ -a+b+c+d = 10 & ...(5) \end{cases}$

$\begin{cases} Eq.1+Eq.2: & 2(a+b+c) = 22 & \Rightarrow a+b+c = 11 & \Rightarrow d = 1 \\ Eq.2+Eq.3: & 2(a+b) = 8 & \Rightarrow \color{#3D99F6}{a+b = 4} & \Rightarrow c = 7 \\ Eq.1+Eq.4: & 2(a+c+d) = 18 & \Rightarrow a+7+1 = 9 & \Rightarrow a = 1 \\ Eq.2+Eq.3: & \color{#3D99F6}{a+b = 4} & \Rightarrow 1+b = 4 & \Rightarrow b = 3 \end{cases}$

$\Rightarrow a\times b\times c \times d = 1\times 3\times 7 \times 1 = \boxed{21}$

a+b+c+d = 12

a+b+c-d = a+b+c+d-2d = 12-2d = 10 -> d=1

a+b-c+d = a+b+c+d-2c = 12-2c = -2 -> c=7

a-b+c+d = a+b+c+d-2b = 12-2b = 6 -> b=3

-a+b+c+d = a+b+c+d-2a = 12-2a = 10 -> a=1

abcd = 1.3.7.1 = 21

turning into augmanted matrix:

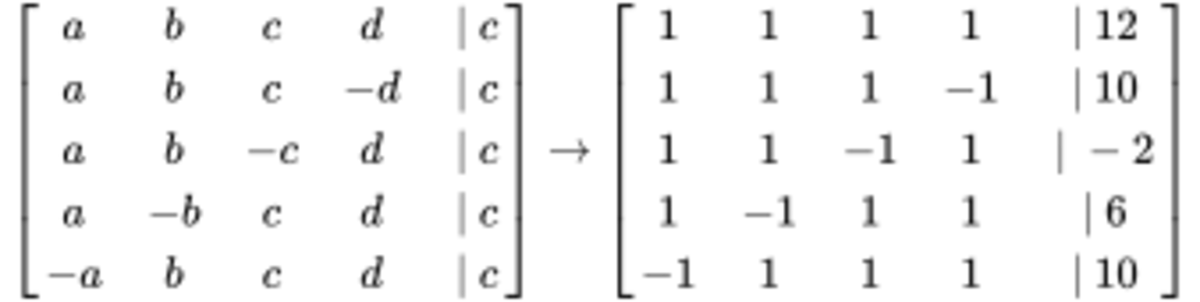
Then subtract row 1 coefficients from all other rows and divide by -2:

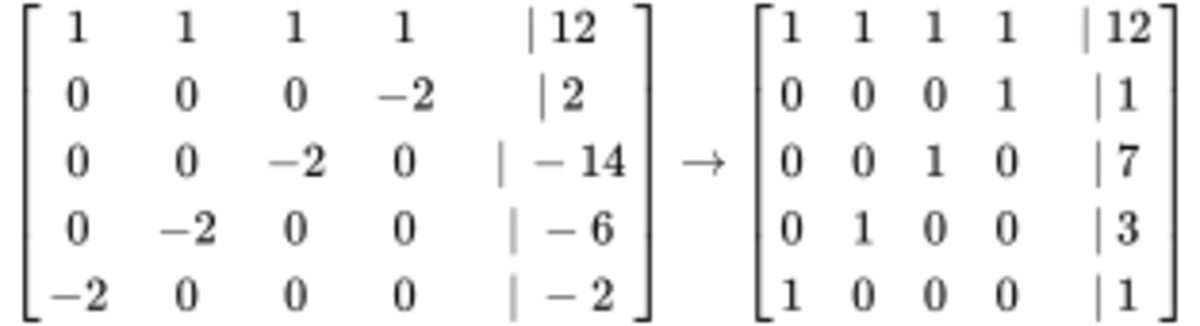
result:

Subtracting eqs. 1 & 2 will create 2d=2, which simplifies to d=1. The same can be done with eqs. 1 & 3 to find c, and so on.

a+b+c+d=12 (1)

a+b+c-d=10 (2)

a+b-c+d=-2 (3)

a-b+c+d=6 (4)

-a+b+c+d=10 (5)

(2) = (5) therefore, a=d.

(3)+(4) = 4 = 2(a+d), a+d=2, thus a=d=1

(2)+(3) = 8, 2(a+b)=8, thus b=3

plug those 3 into any eqn and you get c=7.

a.b.c.d=21

Eq1 minus eq2 same did for eq 4 & 5 and add eq3 into eq 1 get values of all elements a=1,b=3,c=7 & d=1 Now replace values in required eq = axbxcxd=1x3x7x1=21

a+b+c+d = 12 ; then :

to find d, a+b+c = 12-d

a+b+c=10+d -> 12-d = 10+d -> d=1 ;

to find c, a+b+d=12-c

a+b+d=-2+c -> 12-c = -2+c -> c=7 ;

to find b, a+c+d= 12-b

a+c+d = 6+b -> 12-b = 6+b -> b= 3;

to find a, just substitute the rest -> -a+3+7+1=10 -> a=1

abcd= 21

This sistem is impossible (2) + (3) + (4) + (5): 3(a+b+c+d)=24 >> a+b+c+d=8 (1): a+b+c+d = 12 IMPOSSIBLE!

By adding eqns & after solving them,

We get ,a=1,b=3, c=7, d=1

Therefore, axbxcxd= 1×3×7×1=21.