How about rearranging these triangles?

Let $AD=BC=x$ , then applying Pythagorean theorem in $\triangle CBD$ will give: $(3-x)^2+3=x^2\implies x=2$ . $\therefore ?=\sqrt{2^2+3}=\sqrt{7}$ .

The crafty and elegant idea here is to create a rectangle. Let's call it $BDCE$ . Since two diagonals in a rectangle are equal, $BD=DE$ ! We thus have an isosceles triangle, and since the right-angled triangle $BAE$ has a hypotenuse that is twice as long as its side, we realise that the two acute angles in the isosceles triangle are $30^\circ$ each, which gives us another $30-60-90$ triangle! And then we use Pythagoras' Theorem.

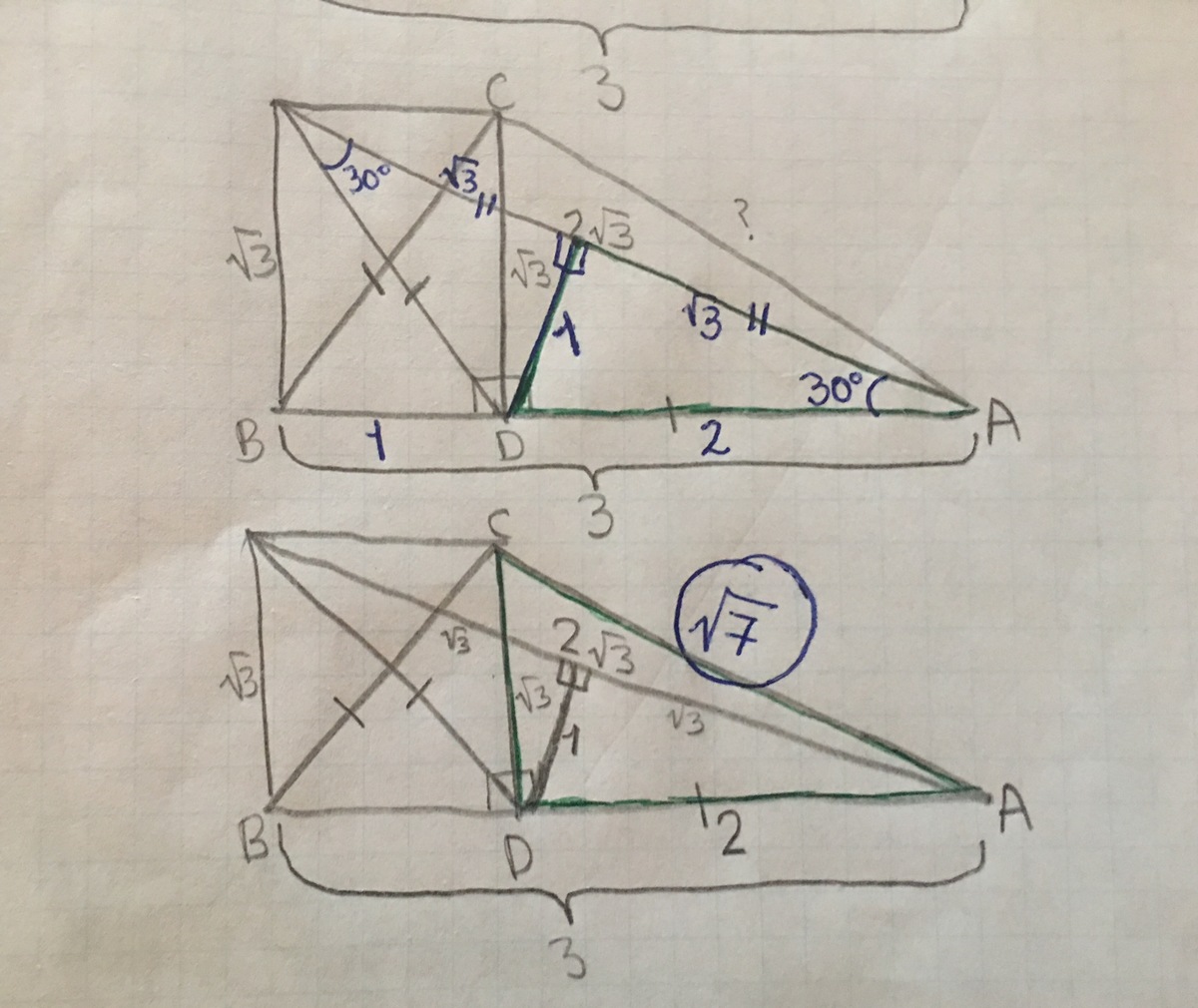
Alternatively, I suggest duplicating $AD$ and putting it over $BD$ to make a big isosceles triangle. That's another way, but my personal preference is to the former.

The triangle $\Delta ADC$ is an right angled triangle.. So, from Pythagorean identity, $AC^2 = CD^2 + AD^2 \Rightarrow AC^2 = 3+AD^2…………(1)$ Now the angle $\angle BAC$ in $\Delta ABC$ is an accute angle.. So, we can apply the expansion of Pythagorean theorem for accute angled triangle.. This is: $BC^2 =AC^2 +AB^2 -2AB.AD$ $\Rightarrow AD^2=AC^2 +(3)^2 -6AD$ ;{Given, $BC=AB$ } $\Rightarrow AD^2 =3 +AD^2 +9-6AD$ {From equation \color\green{(1)} } $\Rightarrow AD =2…… (2)$ So, plugging $AD=2$ in equation \color\green{(1)} , we get... $AC=\sqrt{3+2^2}$ \Rightarrow\boxed{\color\red{AC=\sqrt{7}}}