Ellip-tastic Packing!
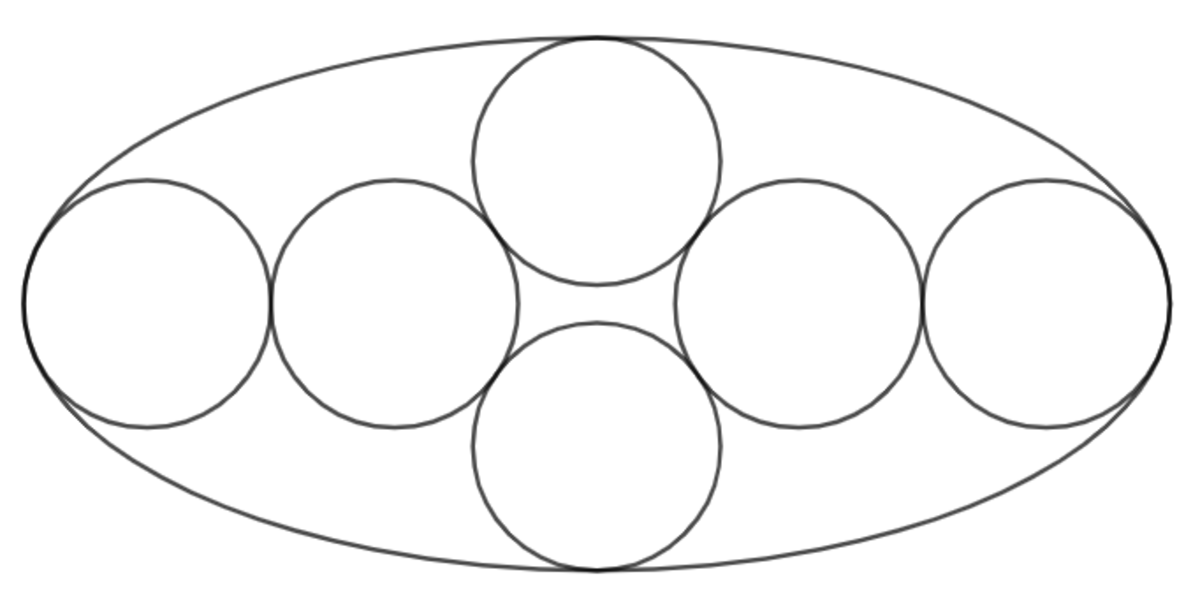
Six tangential identical circles with the maximum possible area are inscribed, such that two of them each share one point of tangency with the endpoint of the major axis, and other two each also share one point of tangency with the endpoint of the minor axis. There exists a unique eccentricity, such that all six circles are positioned symmetrically as shown above.
If the eccentricity is , input as your answer.
The answer is 885562.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let the center of the ellipse be O at the origin, let its semi-major axis be a , let its semi-minor axis be 1 , let the radius of each circle be r , and let the following circles have centers A and B :
Then the ellipse has an equation of a 2 x 2 + y 2 = 1 , and the right-most circle has an equation of ( x − a + r ) 2 + y 2 = r 2 .
Combining these two equations and solving for x gives x = a and x = a 2 − 1 a ( a 2 − 2 a r + 1 ) .
For them to share one point of tangency, x = a = a 2 − 1 a ( a 2 − 2 a r + 1 ) , which solves to r = a 1 .
Also, A B = 2 r , O A = a − 3 r , and O B = 1 − r , so that by the Pythagorean Theorem on △ A O B , ( a − 3 r ) 2 + ( 1 − r ) 2 = 4 r 2 .
Substituting r = a 1 into ( a − 3 r ) 2 + ( 1 − r ) 2 = 4 r 2 solves to a = 3 1 ( − 1 + 3 6 2 − 3 1 8 3 + 3 6 2 + 3 1 8 3 ) .
The eccentricy is then E = a a 2 − 1 ≈ 0 . 8 8 5 5 6 2 3 , which makes ⌊ 1 0 6 E ⌋ = 8 8 5 5 6 2 .