Ellipse Inscribed in a Rectangle
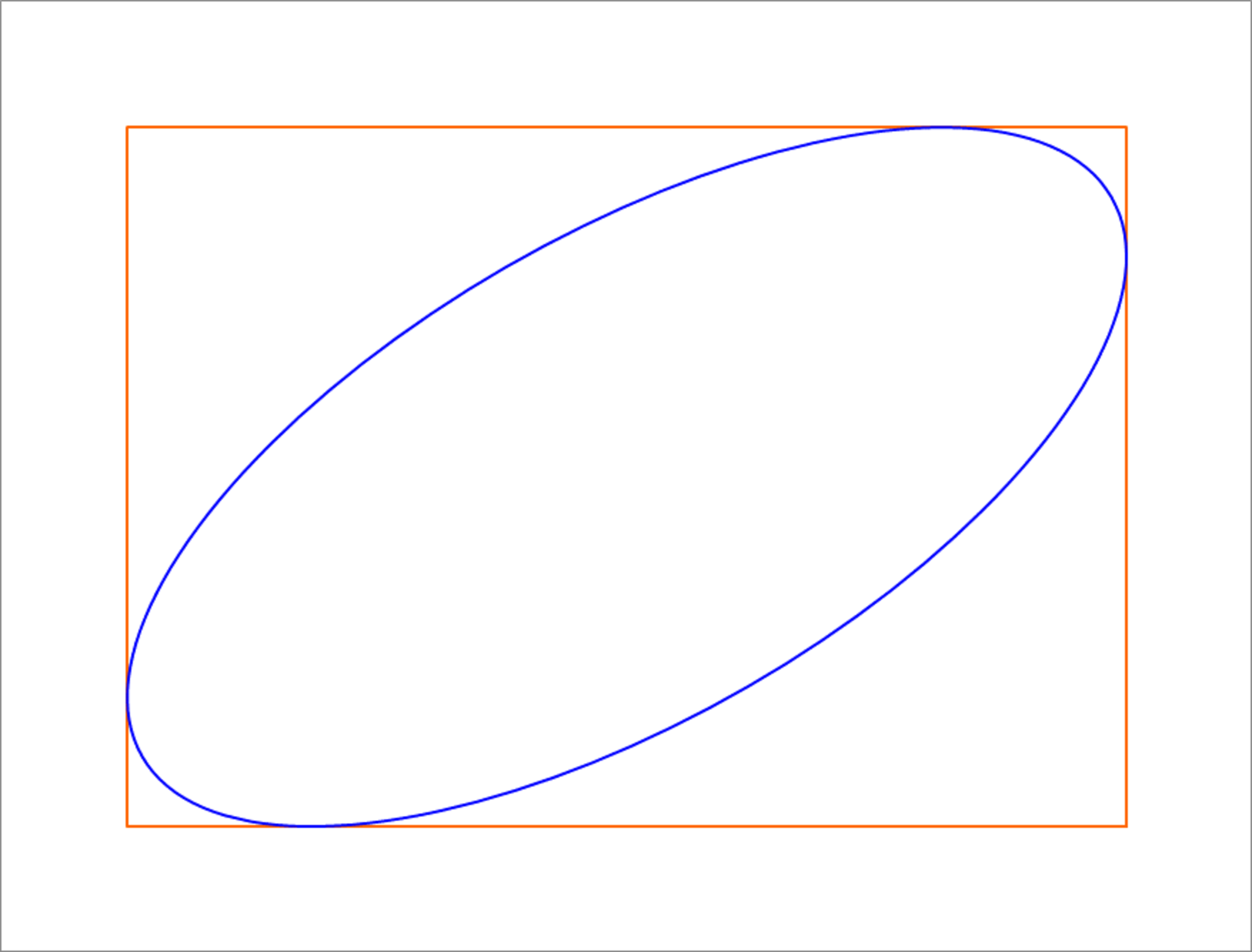
An ellipse in inscribed in a rectangle of dimensions 2 0 × 1 4 , as shown above. If the major axis of the ellipse makes an angle of 3 0 ∘ with the horizontal, find the length of the major axis.
The answer is 22.405.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
For Maxima of of x we set d t d x ( t ) = 0 and get t = − θ thus x m a x = a s i n 2 θ + b c o s 2 θ ....
How did you get that x m a x , y m a x ?
We find equation of tangent to ellipse (orthodox) is
y = m x + / − ( a m ) 2 + b 2
Now 2 parallel tangents are seperated by 14 units (of slope -tan(\pi/6) ) and by 20 (slope of tan(\pi/3) ) .
Thus forming 2 equations in a and b give desired result
The parametric equation of an ellipse rotated by an angle θ counter clockwise is given by
x ( t ) = a cos t cos θ − b sin t sin θ
y ( t ) = a cos t sin θ + b sin t cos θ
The maximum (over t) of both functions, is immediately found to be
x M A X = a 2 cos 2 θ + b 2 sin 2 θ
and
y M A X = a 2 sin 2 θ + b 2 cos 2 θ
From symmetry, the ellipse is centered at the center of the rectangle. Hence,
x M A X = 1 0 and y M A X = 7
Therefore, upon substituting θ in the above equations, we get the system of equations
4 3 a 2 + 4 1 b 2 = 1 0 0
and
4 1 a 2 + 4 3 b 2 = 4 9
Solving this system of equations for a 2 , we obtain,
a 2 = ( 4 3 ( 1 0 0 ) − 4 1 ( 4 9 ) ) / ( 1 6 9 − 1 6 1 ) = 2 2 5 1
Hence,
a = 2 2 5 1
And the major axis length is 2 a = 2 2 2 5 1 = 2 2 . 4 0 5