Empty the vessel

Relevant wiki: Conservation of Energy

Equating potential energy and kinetic energy in a short time period $\Delta t$ when the height of the water is $h$ and the water flow velocity is $v$ :

$\begin{aligned} \Delta m g h & = \frac 12 \Delta m v^2 & \small \color{#3D99F6} \text{where }\Delta m \text{ is mass of water discharged in }\Delta t. \\ \rho \Delta Vgh & = \frac 12\rho \Delta V v^2 & \small \color{#3D99F6} \rho = \text{water density}, \Delta m = \text{ volume of water discharged in }\Delta t.\\ gh & = \frac 12 v^2 \\ \implies v & = \sqrt {2gh} \end{aligned}$

Let the radius of the vessel be $r$ and the cross-sectional area of the outlet be $A$ . Then, we have:

$\begin{aligned} \Delta V = \pi r^2 \Delta h & = Av \Delta t \\ \implies - \frac {dh}{dt} & = \frac {A\color{#3D99F6}v}{\pi r^2} & \small \color{#3D99F6} \text{Note that }v = \sqrt {2gh} \\ & = \frac {A\color{#3D99F6}\sqrt{2gh}}{\pi r^2} \\ - \int \frac {dh}{\sqrt h} & = \int \frac {A\sqrt{2g}}{\pi r^2} dt \\ - 2\sqrt h & = \frac {A\sqrt{2g}}{\pi r^2}t + C \\ \sqrt h & = {\color{#3D99F6}C} - \frac A{\pi r^2}\sqrt{\frac g2} t & \small \color{#3D99F6} \text{where }C \text{ is the constant of integration.} \\ \sqrt 3 & = C & \small \color{#3D99F6} \text{when }t = 0, \ h = 3 \\ \implies \sqrt 0 & = \sqrt 3 - \frac A{\pi r^2}\sqrt{\frac g2} t \\ \implies t & = \frac {\pi r^2}A \sqrt{\frac 6g} \\ & \approx 3160.508 \text{ s} \\ & \approx \boxed{52.7} \text{ min.} \end{aligned}$

Relevant wiki: 2D Kinematics Problem-solving

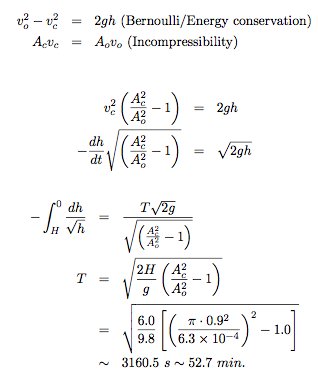
Let the velocity of efflux be $\displaystyle v = \sqrt{2gh} = \frac{dx}{dt}$ .

Now, $\displaystyle A \ dh = a \ dx$ , where $A$ and $a$ are the cross-sectional areas of the vessel and outlet respectively, and $dh$ is the height of liquid in the vessel corresponding to $dx$ in the outlet which decreases in same time $dt$ . Thus,

$\begin{aligned} \frac{A \ dh}{a \ dt} & = \sqrt{2gh} \\ \implies \frac{A \ dh}{a \sqrt{2gh}} & = dt \\ \implies \frac{A}{a \sqrt{2g}} \int_0^h \frac{dh}{\sqrt{h}} & = \int_0^t dt \\ \implies t & = \frac Aa \sqrt{\frac{2h}{g}} \\ \implies \Delta t & = \frac{2A}{a \sqrt{2g}} \left(\sqrt{h_1} - \sqrt{h_2}\right) \end{aligned}$

is the time in seconds required to empty the water from height $h_1 \to h_2$ in the vessel, where $h_1 > h_2$ . Finding the respective values, we have

$\Delta t = \frac{1}{60} \frac{2A}{a \sqrt{2g}} \left(\sqrt{h_1} - \sqrt{h_2}\right) = \frac{1}{60} \frac{2\left(\pi \times 0.9^2 \times 3\right)}{0.00063\sqrt{2 \times 9.8}} \left(\sqrt{3} - \sqrt{0}\right) \approx \boxed{52.7} \ \text{minutes}$

Question: As the water jet impacts onto the ground the vertical component of momentum should decrease in magnitude. Would this "collision" with the ground transmit a pressure through the jet to the outlet to impede the flow of water out from the bottom of the vessel?