Equilateral triangle and two congruent circles!

$\triangle{BMC} \sim \triangle{BOD} \implies \dfrac{\sqrt{3}a}{2\overline{BD}} = \dfrac{a}{2r} \implies \overline{BD} = \sqrt{3}r$

$\implies \overline{DC} = a - \sqrt{3}r = \overline{CE} \implies \triangle{EOC} \cong \triangle{DOC}$ by $s.a.s$

and vertical angles $\angle{OPE} \cong \angle{QPO'} \implies \triangle{EOP} \cong \triangle{PO'Q}$ by $a.a.s$

and $\overline{CQ} \cong \overline{CM} = \dfrac{a}{2} \implies \triangle{CQO'} \cong \triangle{CO'M}$ by $s.a.s$

$\implies$

$A_{\triangle{BMC}} = \dfrac{\sqrt{3}a^2}{8} = A_{\triangle{BOD}} + 2A_{\triangle{DOC}} - A_{\triangle{EOP}} + A_{\triangle{PO'Q}} + 2A_{\triangle{QO'C}}$

$= \dfrac{\sqrt{3}}{2}r^2 + (a - \sqrt{3}r)r + \dfrac{a}{2}r \implies \sqrt{3}a^2 = 12ar - 4\sqrt{3}r^2 \implies$

$4\sqrt{3}r^2 - 12ar + \sqrt{3}a^2 = 0 \implies r = \dfrac{a}{2}(\sqrt{3} \pm \sqrt{2})$ and for

$r = \dfrac{a}{2}(\sqrt{3} + \sqrt{2}) \implies a - \sqrt{3}r < 0$ $\therefore$ we choose $r = \dfrac{a}{2}(\sqrt{3} - \sqrt{2})$

$\implies \dfrac{r}{a} = \dfrac{\sqrt{3} - \sqrt{2}}{2} = \dfrac{\sqrt{\alpha} - \sqrt{\beta}}{\beta} \implies \alpha + \beta = \boxed{5}$ .

Let the equilateral triangle have a side of 2, and it's obvious in a glance that the height is 3r + 2s, with 2s the distance between the two incenters and s the distance between one of them to the intersection point of two pink lines.

s² – r² = [(2 – √3r) – (2 / 1)]² / 4
= (1 – √3r)² / 4
4s² = 7r² – 2√3r + 1

√3 = 3r + 2s
= 3r + √(7r² – 2√3r + 1)
7r² –2√3r + 1 = (√3 – 3r)²
= 9r² – 6√3r + 3
0 = 2r² – 4√3r + 2
= r² – 2√3r + 1
(r – √3)² = 2
r = √3 ± √2
3r < √3
r = √3 – √2
r / a = (√3 – √2) / 2
Answer = 3 + 2 = 5

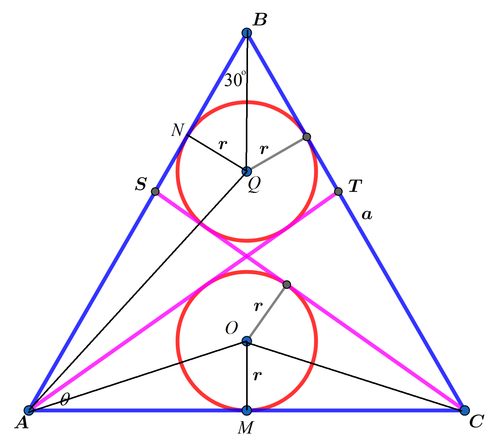
Let the centers of the lower and upper circlers be $O$ and $Q$ respectively, $OM$ and $QN$ be perpendicular to $AC$ and $AB$ respectively, and $\angle TAC = \angle SCA = \theta$ . Using the fact that the line joining a vertex and incenter bisects the vertex angle,

$\begin{aligned} AN + NB & = AB \\ QN \cot \frac {\angle TAB}2 + QN \cot 30^\circ & = AB \\ r \cot \left(30^\circ - \frac \theta 2\right) + r \cot 30^\circ & = a & \small \blue{\text{Let }t = \tan \frac \theta 2} \\ \frac {\sqrt 3 + t}{1-\sqrt 3t}\cdot r + \sqrt 3 r & = a \end{aligned}$

Similarly for $AC$ , we have $\blue{\dfrac {2r}t = a}$ . Then

$\begin{aligned} \frac {\sqrt 3 + t}{1-\sqrt 3t}+ \sqrt 3 & = \frac 2t \\ \frac {\sqrt 3 + t}{1-\sqrt 3t} & = \frac {2-\sqrt 3 t}t \\ 2t^2 - 4\sqrt 3t + 2 & = 0 \\ t^2 - 2\sqrt 3t + 1 & = 0 \\ \implies t & = \sqrt 3 - \sqrt 2 & \small \blue{\text{For }\theta < 90^\circ} \end{aligned}$

Since $\blue{\dfrac {2r}t = a}$ , $\implies \dfrac ra = \dfrac t2 = \dfrac {\sqrt 3-\sqrt 2}2$ . The required answer $\alpha + \beta = 3+2 = \boxed 5$ .