Erk Erk Erk

Calculus Level 5

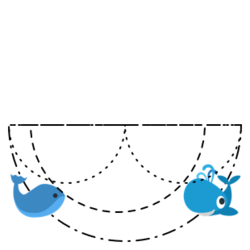Below a depth of about 600 meters, sound travels at a speed that is dependent on the depth of the surface. Sounds actually travel faster the deeper one goes into the ocean, hence sound waves will travel faster by going downwards first.

Suppose that two whales, some distance apart, play at the surface of the sea. One whale emits a cry, which travels toward the other whale along an arc that goes down 7.5 kilometers (without hitting the ocean’s bottom). How long (in seconds) does the cry take to reach the other whale?

Assume that the speed of sound at depth D km is D + 72.5 50 \frac{ D + 72.5 } { 50 } kilometers per second (so the speed changes even at the surface, contrary to the initial statement).

This problem is shared by Gary Antonick .

105 45 10 15

Calvin Lin by Calvin Lin

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Calvin Lin Staff
Apr 21, 2015

This problem deals with finding a geodesic in a non-Euclidean geometry (since the speed varies at different depths). For those who are familiar with the theory, by comparision with the Poincare half plane, you will realize that the geodesics correspond to circles that are 72.5 kilometers above the surface of the ocean.

For a solution, you can refer to the New York Times NumberPlay Blog , that is run by Gary Antonick.

2 Helpful 0 Interesting 0 Brilliant 0 Confused

I defined a term along the lines of refractive index for sound. Since the velocity of sound as a function of depth is given, we get the sound-refractive-index as a function of depth. Then by Fermat's principle of least time, the path of least time is the path followed by light, i.e., one that obeys the laws of refraction.

Then I assumed an arbitary initial angle of incidence and got the slope of the trajectory as a function of depth, finally giving the equation of trajectory of sound for a given angle. Surprisingly it is a circular arc!

Then, I set the initial angle (sin(theta)=0.90625) such that the maximum depth of the trajectory is 7.5 km. This gives the final equation of trajectory. Now what was left was to calculate the time taken to traverse this path. I instead did some manipulations to get an upper bound as 46.6s and the lower bound as 42.3s. This concludes that the answer is 45 seconds, given the options.

Aalap Shah - 6 years, 1 month ago

For the lazy

Pi Han Goh - 6 years, 1 month ago

I somehow got 95.. And so I clicked the closest answer. #Exam skills

Julian Poon - 6 years, 1 month ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...