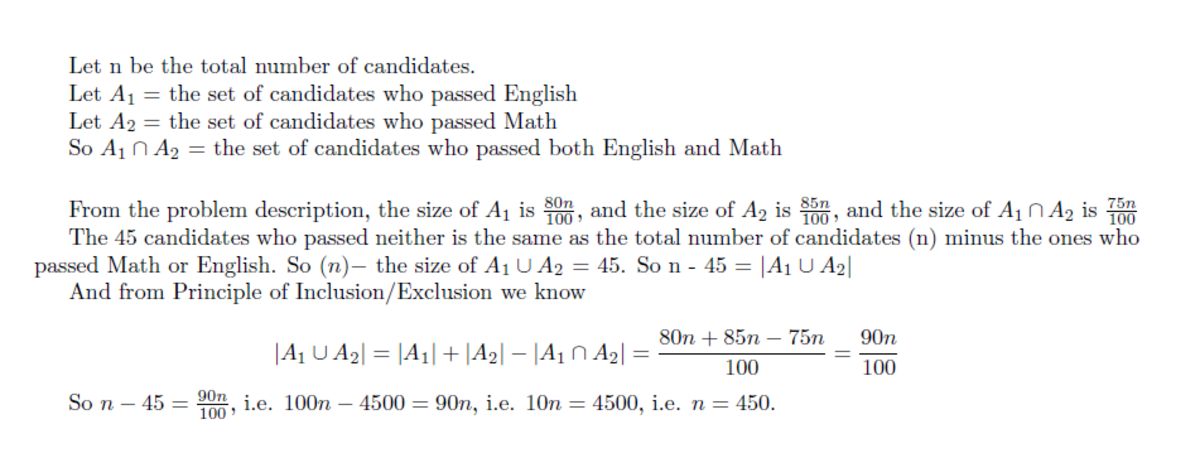
Examination Results

PIE Explanation

Let $|A|$ be the set of people who passed English, let $|B|$ be the set of people who passed Math, and let $|A \cap B|$ be the set of people who passed both.

Using PIE,

$|A|+|B|-|A \cap B|=80+85-75=90$

Thus, 90% passed either the English test, the Math test, or both, meaning that 10% failed both subjects.

Since 10%=45 people, 100% would be 10 times 45

$45*10=\boxed{450}$

75% passed in both the subjects

(80-75)% or 5% passed only in English and (85-75)% or 10% passed only in Mathematics.

Therefore(75+5+10)% or 90% passed in both the subjects and in one subject.

Or, 10% failed in both subjects

From the question,

10% of total candidates=45

Total candidates=450

Because number of candidates failed in both is consisted in both examinees have failed in in English and Mathematics.
The percentage number of candidates failed in both is equal to: $[200-(80+85)]-(100-75)=10 (\%)$ Number of candidates is: $10 \times 45 = \boxed{450}$

So First, we have to find percentage of examinees that passed on English or Math,

80%+85%-75% = 90%

So there is 10% that not passed the exam, and we know the number is 45 so 10% = 45 candidates Just multiply by 10 to get 100%

45*10 = 450

(80 - 75) = 5% passed in English only.
(85 - 75) = 10 % passed in Mathematics only.
75% passed in both.
So (75 + 5 + 10) = 90 % passed in at least one of them.
(100 - 90) = 10% failed in both.
Since 45 = 10% , 45/(10%) = 100% = 450 students in total.

But wait ...... Now that we know the total number of students, we can get exact numbers for each group.
450 * 10% = 45 passed in Mathematics only.
450 * 10% = 45 failed in both.
So far, so good.
450 * 5% = 22.5 passed in English only. (?????)
450 * 75% = 337.5 passed in both. (?????)

The number students must always be an integer, so you can't have half a student passing a test.

It would have been alright if it was about red and blue flower along a stretch, since you can have 22,5 meters with only red flowers and 337.5 meters with a mixture.

P(EUM)=0.8+0.85-0.75=0.9 so 90% of students are passed in either of subjects or both subjects. Hence 10% of students were failed in both subjects which is equal to 45 students. So number of students who passed is 90%=405 students. Therefore total number of students are 405+45=450.

Each person falls into one of four sets:

• those that pass both E and M (75%)
• those that pass E but fail M (80%-75%=5%)
• those that fail E but pass M (85%-75%=10%)
• those that fail both E and M must be the rest: 100%-75%-5%-10%=10%

If the 45 candidates who failed constitute 10% of all candidates, the total number of candidates was 450.

Side note: 75% and 85% would imply 337.5 and 382.5 people, so the given percentages must have been rounded figures. And assuming the percentage figures were rounded to the nearest integer, this in turn implies that our estimate has an uncertainty of about 5 people.

This question is duplicate. I already answered the same. It would be good find the duplicate and (computerly) reunify the answers.

First Step : Draw 2 Circle over lapping in the middle (As you would in a Venn Diagram) Second Step : Fill in all known Information Math = 85% English = 80% Both = 75% Failed both = x% = 45 Third Step : Use the Principle of Inclusion and Exclusion. For Math : Since 75% passed both test, and there are 85% that passed Math, We can conclude that the percentage of people that only passed in math is 10% Do the same to English, and you get 5%.

And all the percentage 75%+10%+5%=90% So 90% of the participant passed the test. And we know there are 45 people that failed, and these 45 people are the 10% of people that didn't make it. 10/45=100/z Z = 450 people.