Extending the model
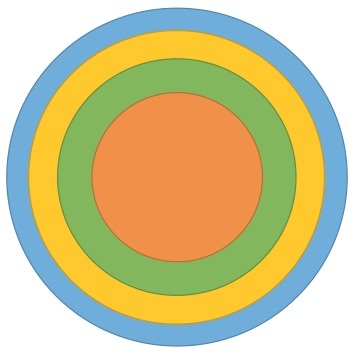 All circles in the figure have the same center. The area of region between the circles is equal to the area of the smaller circle ( one in orange). If we extend the model to 100 circles, what is
where
is the radius of the
circle.
All circles in the figure have the same center. The area of region between the circles is equal to the area of the smaller circle ( one in orange). If we extend the model to 100 circles, what is
where
is the radius of the
circle.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The area of a circle is proportional to its squared radius.
Proof for four circles :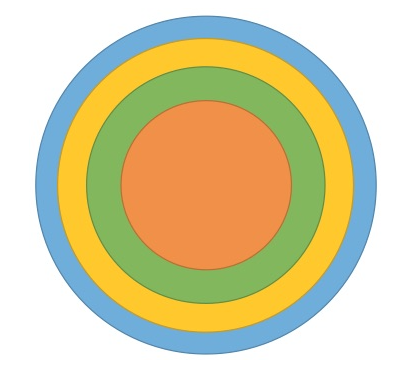 π
R
2
2
−
π
R
1
2
=
π
R
1
2
+
π
R
2
2
−
π
R
1
2
=
π
R
1
2
+
π R 3 2 − π R 2 2 = π R 1 2 +
π R 4 2 − π R 3 2 = π R 1 2 ⟹ π R 4 2 − π R 1 2 = 3 π R 1 2 ⟹ R 4 2 = 4 R 1 2 ⟹ R 1 R 4 = 2
The same model can be extended to 1 0 0 circles with the result that the square root of 1 0 0 = 1 0