Failed to escape 'n caught by the Moon
Imagine, for now, the Sun 'n the Venus and the Mars 'n the Uranus, all have gone. What's left behind is our Earth 'n Moon. And they have gone stationary.

As you see here, the mass of earth and the mass of the moon is . And the distance between them ( Center 2 Center ) measures , as it should be. But you know, something must change, the radius of the earth became but the radius of the moon is, as usual, .
Now we wanna send a rocket from the earth's surface , and then we want it to land on the moon surface . Calculate the least velocity ( in ) it must be given.
Assume the rocket to be a point object and that it travels along the line joining the centers of the planets.
Given :
HInt: The answer isn't 1801, it's more...
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
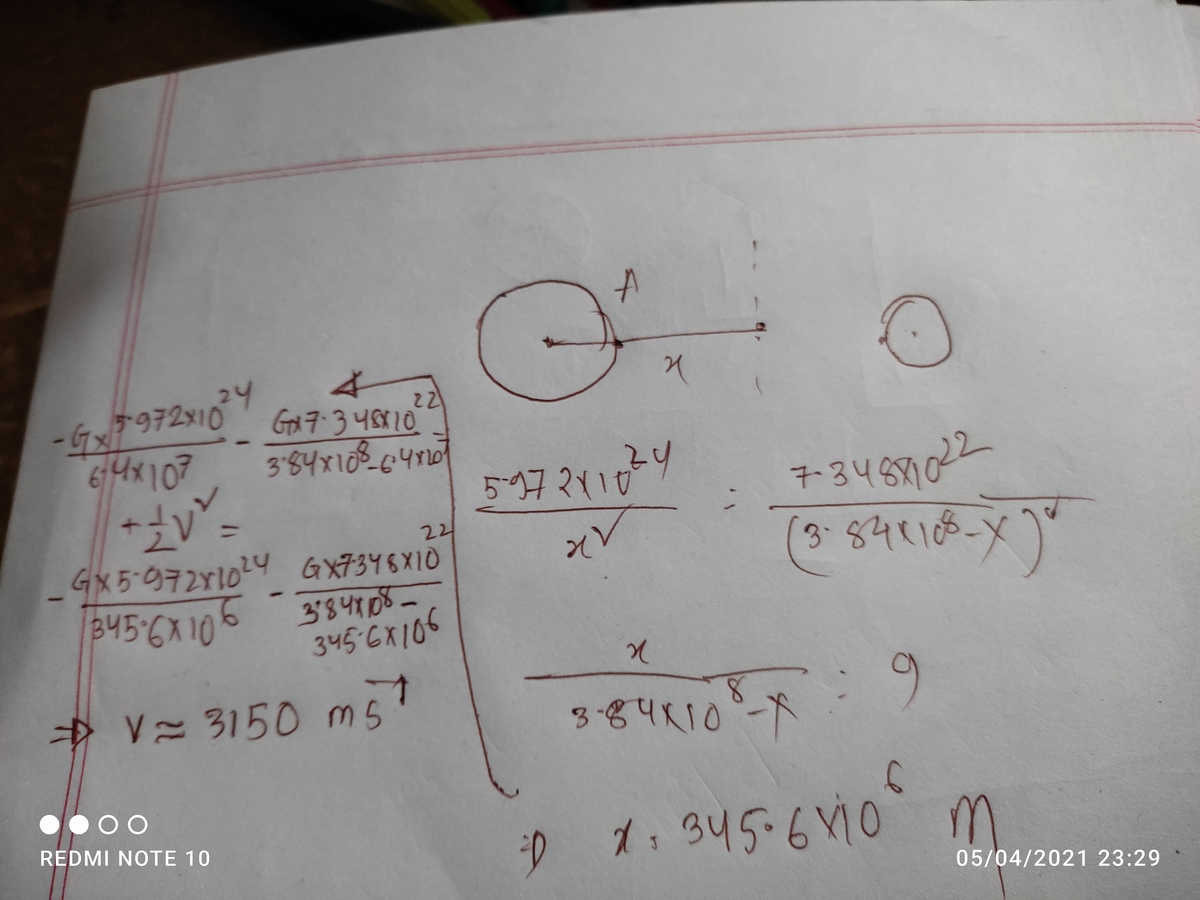
It makes it easier when we use the concept of diverging space and replace force with fluid flow. The situation stands; Earth and the Moon acting like sinks. We only need to travel where the flow is calm(in this case 3.45*10^8, where the net force is 0 N) after that the inertia will give a little push and the sink at the moon will pull me towards the moon all by it self. By calculating the work done till the neutral point and equating it with Kinetic Energy we get the velocity which is around 3150 m/s