Fanning Fans
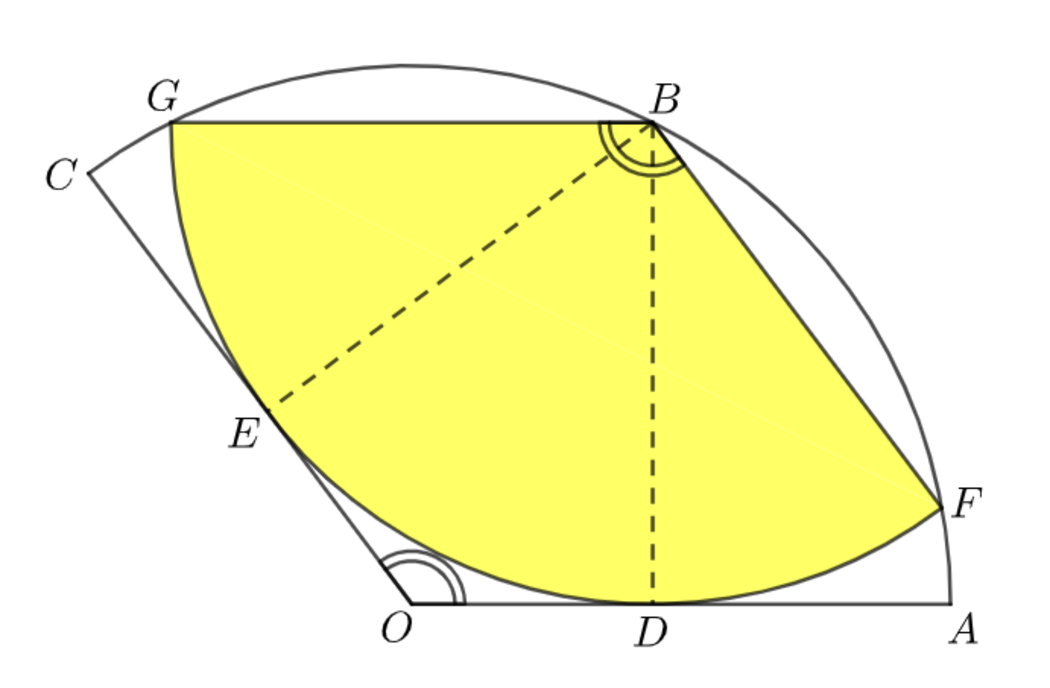
The circular sector is symmetrically inscribed in the large circular sector , such that they share five points of tangency and . Two quarter circles and each share four points of tangency with sector . It can be shown that the area ratio of sector to sector can be expressed as , where and are coprime positive integers. Find the value of .
The answer is 9.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The figure is symmetrical about the straight line O B . Therefore O B bisects both ∠ A O C and ∠ F B G . Since ∠ A O C = ∠ F B G = θ , ∠ A O B = ∠ G B O = 2 1 θ and A O ∥ B G . Let the radii of sector F B G and sector A O C be r and 1 respectively. Then we note that \BG=r) and O B = O G = 1 . Therefore △ O B G is isosceles and ∠ B G O = ∠ G B O = 2 1 θ . Also B G = r = 2 cos 2 θ . We also note that B D = r = sin 2 θ . So we have:
sin 2 θ ⟹ tan 2 θ r = 2 cos 2 θ = 2 = sin 2 θ = 5 2
The ratio of areas of the two sectors [ A O C ] [ F B G ] = 1 2 r 2 = 5 4 and the required answer a + b = 4 + 5 = 9 .