Filtered Curve vs. Original (Part 3)
Two discrete curves are defined as follows:
Δ θ = 1 0 0 0 π α = 0 . 9 9 5 k = 0 , 1 , 2 , 3 . . . . 1 0 0 0 θ k = k Δ θ x k = cos ( θ k ) y k = sin ( θ k ) y k ′ = α y k − 1 ′ + ( 1 − α ) y k y 0 ′ = 0
Scatter plots of ( x k , y k ) and ( x k , y k ′ ) are shown as smooth lines for display purposes.
The k subscript denotes the present value of the variable, and the k − 1 subscript denotes the previous value of the variable. The quantity y ′ is the result when y is passed through an infinite-impulse-response filter.
What is the ratio of the blue-shaded area to the tan-shaded area?
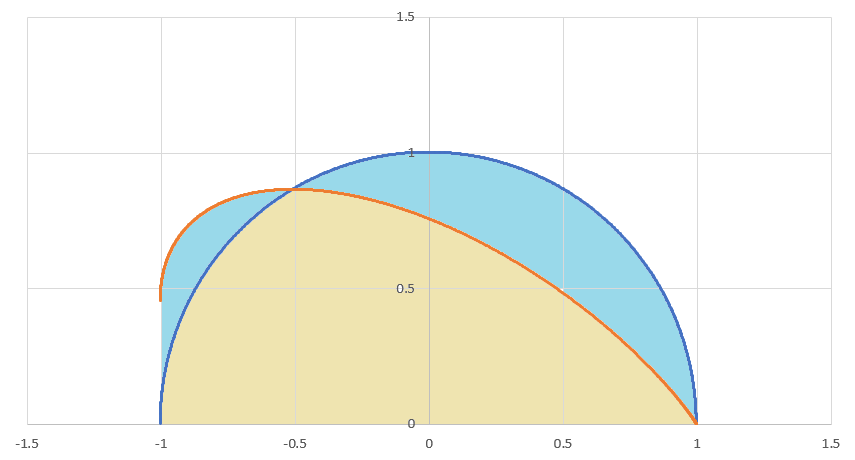
The answer is 0.417.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
0 solutions
No explanations have been posted yet. Check back later!