find alpha angle in the triangle

Geometry Level 2


The answer is 40.

Aly Ahmed by Aly Ahmed , 34, Egypt

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

3 solutions

Aly Ahmed
May 7, 2020

{: .cente||

1 Helpful 0 Interesting 0 Brilliant 0 Confused

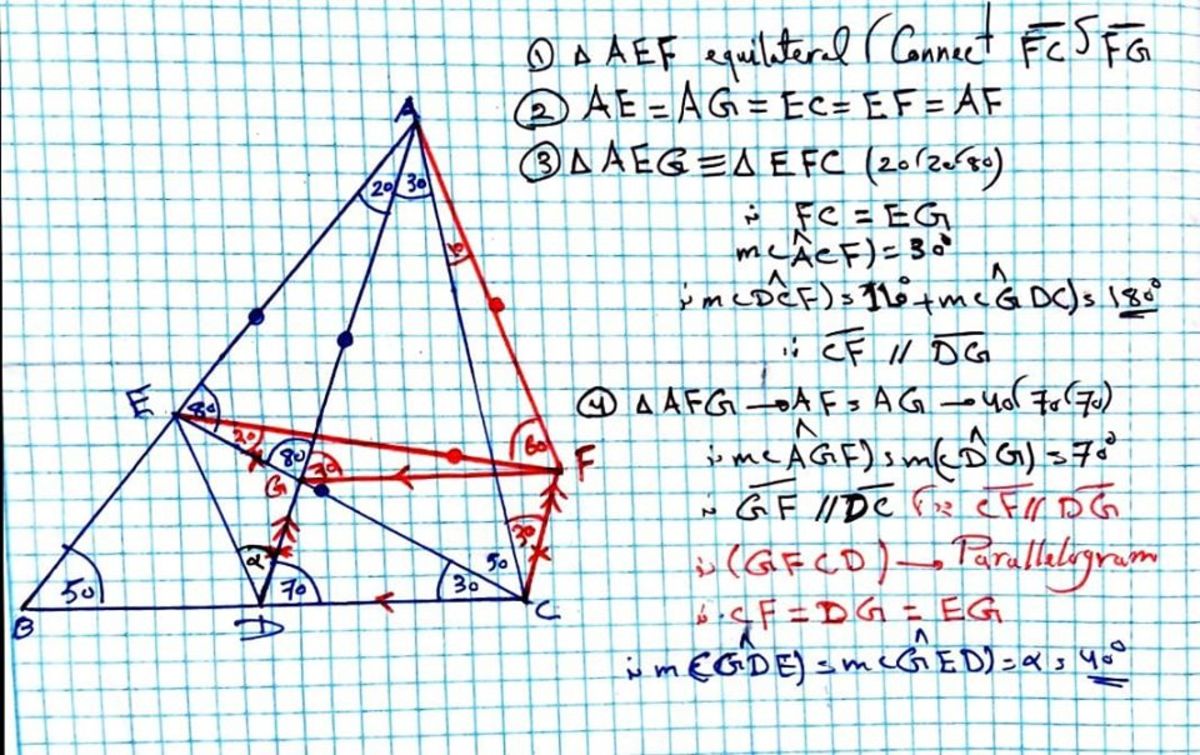
nice approach!

nibedan mukherjee - 1 year, 1 month ago
Trupal Panchal
May 7, 2020

i tried very hard but won't able to find the method to solve for alpha then i actually draw it and measure the alpha which is 40.

0 Helpful 0 Interesting 0 Brilliant 0 Confused

It's easy to get sin ⁡ α sin ⁡ 20 ° = sin ⁡ ( 70 ° + α ) sin ⁡ 30 ° ⟹ sin ⁡ α = 2 sin ⁡ 20 ° sin ⁡ ( 70 ° + α ) ⟹ cos ⁡ 40 ° sin ⁡ α = sin ⁡ 40 ° cos ⁡ α ⟹ tan ⁡ α = tan ⁡ 40 ° ⟹ α = 40 ° \dfrac {\sin α}{\sin 20\degree}=\dfrac {\sin (70\degree+α)}{\sin 30\degree}\implies \sin α=2\sin 20\degree\sin (70\degree+α)\implies \cos 40\degree\sin α=\sin 40\degree\cos α\implies \tan α=\tan 40\degree\implies α=\boxed {40\degree} .

0 Helpful 0 Interesting 0 Brilliant 1 Confused

your math is wrong dude, the third step should be 'cos(50) sin (alpha) = sin (50) cos (alpha)' because 70-20=50 not 40.

tell me if i miscalculated. thank you.

trupal panchal - 1 year, 1 month ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...