Find angle in two triangles
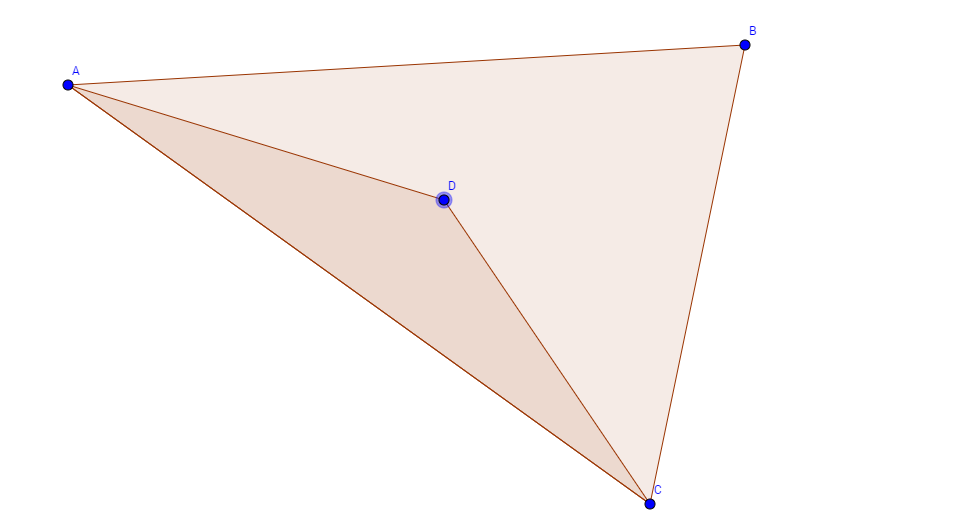
∠ D A C = 2 0 ∘ , ∠ D C A = 3 0 ∘ , ∠ A B C = 5 0 ∘ , A D = B C .
Find the measure of ∠ D C B in degrees.
The answer is 70.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
 c
o
n
s
t
u
c
t
a
p
o
i
n
t
E
w
h
e
r
e
A
E
=
C
B
,
E
C
=
A
B
a
n
d
A
E
/
/
B
C
,
E
C
/
/
A
B
⇒
A
E
C
B
i
s
/
/
g
r
a
m
.
⇒
∠
A
B
C
=
A
E
C
=
5
0
°
∠
A
D
C
=
1
8
0
°
−
2
0
°
−
3
0
°
=
1
3
0
°
∠
A
D
C
+
∠
A
E
C
=
1
8
0
°
⇒
A
D
C
E
i
s
c
o
n
c
y
c
l
i
c
∵
A
D
=
B
C
=
A
E
⇒
A
C
D
=
A
C
E
=
3
0
°
⇒
E
A
C
=
1
8
0
°
−
3
0
°
−
5
0
°
=
1
0
0
°
A
C
B
=
E
A
C
=
1
0
0
°
⇒
D
C
B
=
1
0
0
°
−
3
0
°
=
7
0
°
c
o
n
s
t
u
c
t
a
p
o
i
n
t
E
w
h
e
r
e
A
E
=
C
B
,
E
C
=
A
B
a
n
d
A
E
/
/
B
C
,
E
C
/
/
A
B
⇒
A
E
C
B
i
s
/
/
g
r
a
m
.
⇒
∠
A
B
C
=
A
E
C
=
5
0
°
∠
A
D
C
=
1
8
0
°
−
2
0
°
−
3
0
°
=
1
3
0
°
∠
A
D
C
+
∠
A
E
C
=
1
8
0
°
⇒
A
D
C
E
i
s
c
o
n
c
y
c
l
i
c
∵
A
D
=
B
C
=
A
E
⇒
A
C
D
=
A
C
E
=
3
0
°
⇒
E
A
C
=
1
8
0
°
−
3
0
°
−
5
0
°
=
1
0
0
°
A
C
B
=
E
A
C
=
1
0
0
°
⇒
D
C
B
=
1
0
0
°
−
3
0
°
=
7
0
°
Excellent problem and solution! (+1)
smart way of answering this question
Applying the law of sines to the triangle ACD, we obtain
(1) sin ( ∠ D C A ) A D = sin ( ∠ A D C ) A C ,
or, since ∠ D C A = 3 0 ∘ and ∠ A D C = 1 8 0 ∘ − ∠ D A C − ∠ D C A = 1 3 0 ∘ ,
(2) A C A D = sin 1 3 0 ∘ sin 3 0 ∘ .
Analogously, in the triangle ABC we have
(3) sin ( ∠ B A C ) B C = sin ( ∠ A B C ) A C ,
or, since ∠ A B C = 5 0 ∘ and ∠ B A C = 1 8 0 ∘ − ∠ A B C − ∠ A C B = 1 3 0 ∘ − ∠ A C B ,
(4) A C B C = sin 5 0 ∘ sin ( 1 3 0 ∘ − ∠ A C B ) .
Since A D = B C , it follows from (2) and (4) that
(5) sin 1 3 0 ∘ sin 3 0 ∘ = sin 5 0 ∘ sin ( 1 3 0 ∘ − ∠ A C B ) .
One can simplify (5) by noticing that sin 1 3 0 ∘ = sin 5 0 ∘ , so that sin 3 0 ∘ = sin ( 1 3 0 ∘ − ∠ A C B ) , from which follows ∠ A C B = 1 0 0 ∘ . Finally, we obtain the desired result by subtracting ∠ D C A from ∠ A C B :
(6) ∠ D C B = ∠ A C B − ∠ D C A = 1 0 0 ∘ − 3 0 ∘ = 7 0 ∘ . □