Find Max + Min

The equation $x^2+y^2-14x-6y=6$ defines a circle centered at $(7,3)$ . The linear function $f(x,y)=3x+4y$ will attain its constrained extrema on opposite points on this circle, $(7+a,3+b)$ and $(7-a,3-b)$ , so that the sum of these two extrema is $2f(7,3)=\boxed{66}$ .

$\begin{aligned} x^2+y^2-14x-6y & = 6 \\ (x-7)^2 + (y-3)^2 & = 6+49+9 = 64 \end{aligned}$

$\implies (x-7)^2 + (y-3)^2 = 8^2$ a circle with center at $(7,3)$ and a radius of $8$ . We can substitute

$\begin{cases} x - 7 = 8\cos \theta & \implies x = 8 \cos \theta + 7 \\ y - 3 = 8\sin \theta & \implies y = 8 \sin \theta + 3 \end{cases}$

Then,

$\begin{aligned} 3x+4y & = 24 \cos \theta + 21 + 32\sin \theta + 12 \\ & = 40 \sin \left(\theta + \tan^{-1} \frac 34\right) + 33 \\ \implies \max (3x+4y) & = 40 + 33 & \small \color{#3D99F6} \text{when } \sin \left(\theta + \tan^{-1} \frac 34\right) \text{ is maximum or }= 1 \\ \implies \min (3x+4y) & = -40 + 33 & \small \color{#3D99F6} \text{when } \sin \left(\theta + \tan^{-1} \frac 34\right) \text{ is minimum or }= -1 \end{aligned}$

Therefore $\max (3x+4y) + \min (3x+4y) = \boxed{66}$ .

We look at it from a geometrical point of view ....... The equation just boils down to a equation of circle with center $(7,3)$ and with radius $8$ units . Now it is evident that the maximum and minimum value $c$ of $3x+4y=c(say)$ would be for the situations for which the line is tangent to this circle. In other words we are looking for the maximum and minimum value of c for which the parallel straight lines become tangent to the circle. Now we apply the fact that the perpendicular distance of the center from the line would be equal to the radius.......

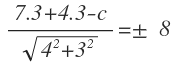
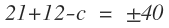
which gives the values of $c$ = $-7$ and $73$ . Adding them we get our answer as $66$

$x^2+y^2-14x-6y=6\implies (x-7)^2+(y-3)^2=8^2\\ \implies x=7+8\cos(\theta), y=3+8\sin(\theta)\\ \implies 3x+4y=(21+24 \cos(\theta))+(12+32\sin(\theta))\\ =33+24\sqrt{2}\sin(\theta+\frac{\pi}{4})+8\sin(\theta)$

This value is maximum for $\theta =\frac{\pi}{4}$ and minimum for $\theta =\frac{-3\pi}{4}$ .

Therefore maximum value will be $33+24\sqrt{2}+4\sqrt{2}=33+28\sqrt{2}$ and minimum will be $33-28\sqrt{2}$ .

Hence the sum is $33+28\sqrt{2}+33-28\sqrt{2}=\boxed{66}.$