Find my xy

We have $3^x=5$ , increasing the power by $y$ on both side we have

$3^{xy}=5^y=9$ .

Equating $3^{xy}=3^2$ , we obtain $xy=2$

We have ${ 3 }^{ x }=5 , 5^{ y }= 9\\ \implies x=\log_{3}{5},y=\log_{5}{9}=2\log_{5}{3}\\ \implies xy=2\log_{3}{5}\cdot\log_{5}{3} \\ =2\cdot\dfrac{\log5}{\log3}\cdot\dfrac{\log3}{\log5} =2.$

Note : $\large{\log_{\color{#20A900}{a}}{\color{#3D99F6}{b}}=\dfrac{\log\color{#3D99F6}{b}}{\log\color{#20A900}{a}}} \quad\quad\quad\quad \text{Base-changing rule}$

Here, we use the change of base formula. By solving for x and y, we get y=base 5 log 9 and x=base 3 log. We can now solve for xy:

xy=(base 5 log 9)(base 3 log 5) = $\frac{(log 9)(log 5)}{(log 5)(log 3)}$ = $\boxed{2}$

Note:

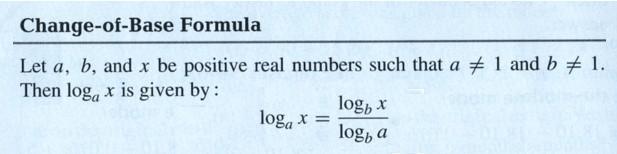
Log both sides of the equations. Therefore,we achieve the 2 equations as follows: lg3^x=lg5 and lg5^y=lg9 From here,we can deduce that x=lg5/lg3 and y=lg9/lg5 Multiplying both x and y together,we end up with the answer of 2.

We can rewrite $3^{x}=5$ as $3^{x}=5^{1}$ and $5^{y}=9$ as $5^{y}=3^{2}$ . Then, for both equations to be the same make $x=2$ and $y=1$ . Then $xy=1\times2=2$ .

Being that log_{a}b=c is equivalent to a^{c}=b , we can calculate the values of x band y , therefore calculating xy which rounded to the closest integer equals 2.

There is a mistake in the given question, it should be 3^x=5 and 5^y=9