Find the angle

SOLUTION 1: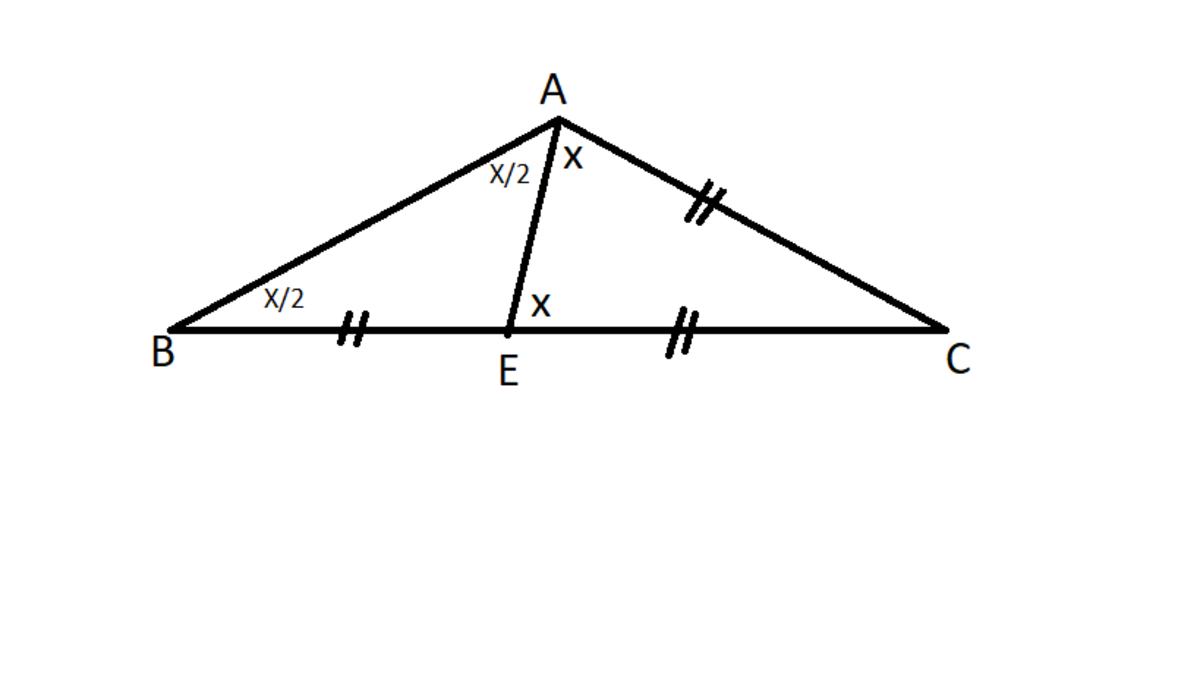 OKAY! the problem may seem easy at first sight , but trust me,it took me 3 hours to reach the solution!!now,lets move towards to the solution.it is given that the segment BC is twice segment AC.let us draw another line(the median) that touches BC at point
E.From the picture,it is clear that AC=CE=BE.However.in triangle AEC, AC=CE. thus it is an isosceles,and the shown angles are congruent.Let us determine them as X. angleAEC=X is an exterior angle of triangle AEB. so, ∠X=∠ABE+∠BAE
=∠B+(∠A-∠X)
=∠B+∠A-∠X
=∠B+3∠B-∠X
=4∠B-∠X
so,2∠X=4∠B
or, ∠B= $\frac{∠X}{2}$ . ∠X=∠B+∠BAE
or, ∠X= $\frac{∠X}{2}$ +∠BAE . NOW,∠BAE= $\frac{∠X}{2}$ .SO, AEB is an isosceles.AND,BE=AE=AE=EC=AC.so,triangle AEC is equilateral and ULTIMATELY we find X is equal to 60 degree.BUT,angle A=X+ $\frac{∠X}{2}$ =60+30= 90

SOLUTION 2(ALTERNATIVE): Simply apply sine law ,
$\frac{AB}{sinC}$ = $\frac{BC}{sinA}$ = $\frac{AC}{sinB}$
Taking last two ratios,
$\frac{sinA}{sinB}$ = $\frac{AC}{BC}$ =2
=>sin3B=2sinB
=>3sinB-4(sinB)^3=2sinB
=>3-4(sinB)^2=2 [dividing both sides by sinB, as sinB≠ 0]
=>(sinB)^2=1/4
=>sinB=1/2=sin30
so, ∠B=30
so, ∠A=3∠B=3x30=90 degree.

Let $\angle B = \theta$ and $AC=1$ ; then $\angle A = 3\theta$ and $BC = 2$ . Using sine rule ,

$\begin{aligned} \frac {\sin \angle A}{BC} & = \frac {\sin \angle B}{AC} \\ \frac {\sin 3\theta}2 & = \frac {\sin \theta}1 \\ \sin 3\theta & = 2\sin \theta \\ 3\sin \theta - 4\sin^3 \theta & = 2\sin \theta \\ \sin \theta - 4\sin^3 \theta & = 0 \\ \sin \theta( 1 - 4\sin^2 \theta) & = 0 \end{aligned}$

$\implies \sin \theta = 0 \implies \theta = 0^\circ \implies \angle A = 3\theta = \color{#D61F06} 0^\circ \quad \small \color{#D61F06} \text{Not acceptable}$

$\implies \sin \theta = \pm \frac 12 \implies \begin{cases} \theta = 30^\circ & \implies \angle A = 3\theta = \color{#3D99F6} \boxed{90^\circ} & \small \color{#3D99F6} \text{Acceptable} \\ \theta = 120^\circ & \implies \angle A = 3\theta = \color{#D61F06} 360^\circ & \small \color{#D61F06} \text{Not acceptable} \end{cases}$