Find the angle $\angle NFL$

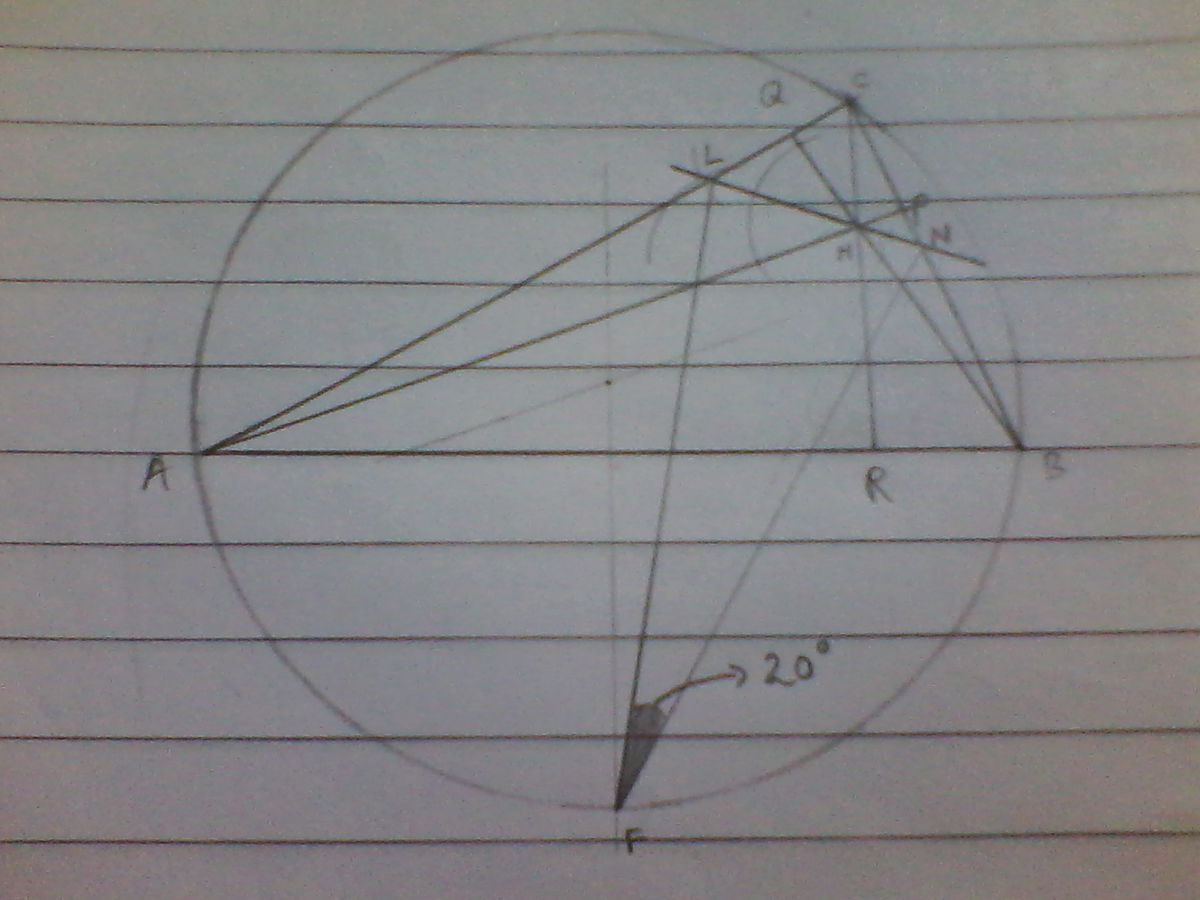
Extend $AP$ and $BQ$ to hit the circumcircle at $S$ and $T$ , respectively. It is well-known that $\angle ABC = \angle CBS$ . Thus, we have $N$ is the $\textbf{incenter}$ of $\triangle BHS$ . Thus, $SN$ is the angle bisector of $\angle BSA$ .

Since $\angle BSA$ substends arc $AB$ and $F$ is the midpoint of this arc, we have that $SN$ hits $F$ . Similarly, $TL$ hits $F$ . Thus, $\angle NFL = \frac{\widehat{ST}}{2} = 180^{\circ} - 2\angle C = \boxed{20^{\circ}}$

Say AC=1,then CR=1/2,AR=√3/2

We can put coordinate system in A with x-axis as AB

Now we know coordinates of points

A(0,0),C(√3/2,1/2),B(AB,0)

AB=AR+RB=√3/2+CR×ctg(70°) so

B((ctg(70°)+√3)/2,0) Now

AQ=AB×sin(<ABQ)=(√3+ctg(70°)×√3/4

Now we can find coordinates of Q

x(Q)/AR=AQ/AC so

x(Q)=AQ AR=3/8 (√3+ctg(70°))

y(Q)=AQ sin(<QAR)=√3/8 (√3+ctg(70°)

AL/LQ=AH/QH

Angle bisector divide AQ in yhe ratio of sides AH:HQ

We can calculate easily that

<AHQ=80° and QH=AH×cos(80°)

So LQ/AL=cos(80°) and now

LQ/AL+1=(LQ+AL)/AL=AQ/AL

So Al=AQ/(1+cos(80°)=AQ/(2cos²(40°))

Also AL/AQ=X(L)/X(Q)=Y(L)/Y(Q)

So Y(L)=Y(Q)/(2cos²(40°)) and

X(L)=X(Q)/(2cos²(40°))

So Now we have coordinates of point L

Similiar PN/NB=HP/HB=cos(80°) and

PB/NB=1+cos(80°)=2cos²(40°)

NB=PB/(2cos²(40°)) and

PB=AB×cos(70°)

X(N)=AB-NB×cos(70°) so

X(N)=AB×(1-cos²(70°)/(2cos²(40°))

Y(N)=NB×sin(70°)

Now we know coordinates of N

X(F)=AB/2

If O is center of that circle above ABC

Y(O)=√(R²-(AB/2)²)

Y(F)=Y(O)-R

So N(0.94355507604774,0.286988976129769)

F(0.52400526045877,-0.43969262078596)

L(0.77331840369432,0.386659201547161)

So vectors (FN) and (FL) have coordunates

FN(0.4235024714597,0.72670823581252)

FL(0.14570812182492,0.82637846123912)

So scalar product(inner product)

(FN)•(FL)=FN×FL×cos(<NFL)

<NFL=arccos((FN)•(FL)/(FN×FL))

So <NFL~20.232° with my scientific calculator app

Because it must be integer <NFL=20°

Or we can prove that expression we have

Is cos(20°) after some manipulation

I use 12digits,and error was 0.232°

I thought it would be smaller error

But |cos(20°)-cos(20.232°)|~0.001

I constructed it !!

Simple chasing yields that <CAP=10°, <ACR=60°, <BCR=20°. Now join C,F ; A,F ; B,F. Let CF meet LN at the point X. Since arc AF= arc BF, <ACF=<BCF. Simplifying that with the aforementioned values yields that <ACF=<LCX=40°. Observing ∆AHQ yields that <AHQ=80°. Thus <LHQ=40°. Thus <QLH=50°. This implies that ∆CXL is right angled and ∆CLN is isosceles. So we get that XL=XN and XF is perpendicular to LN. Therefore ∆LNF is either equilateral or isosceles. Extend AP upto Y such that Y intersects the circumcircle of ∆ABC. So HP=PY and NP is perpendicular to HY. So we get that ∆NHY is also isosceles. Now simply we can get that <NHY=NYH=40°. Again considering the outside angle <LNF of ∆NHY yields that <LNF=40+40=80°. So finally <NFL=20°