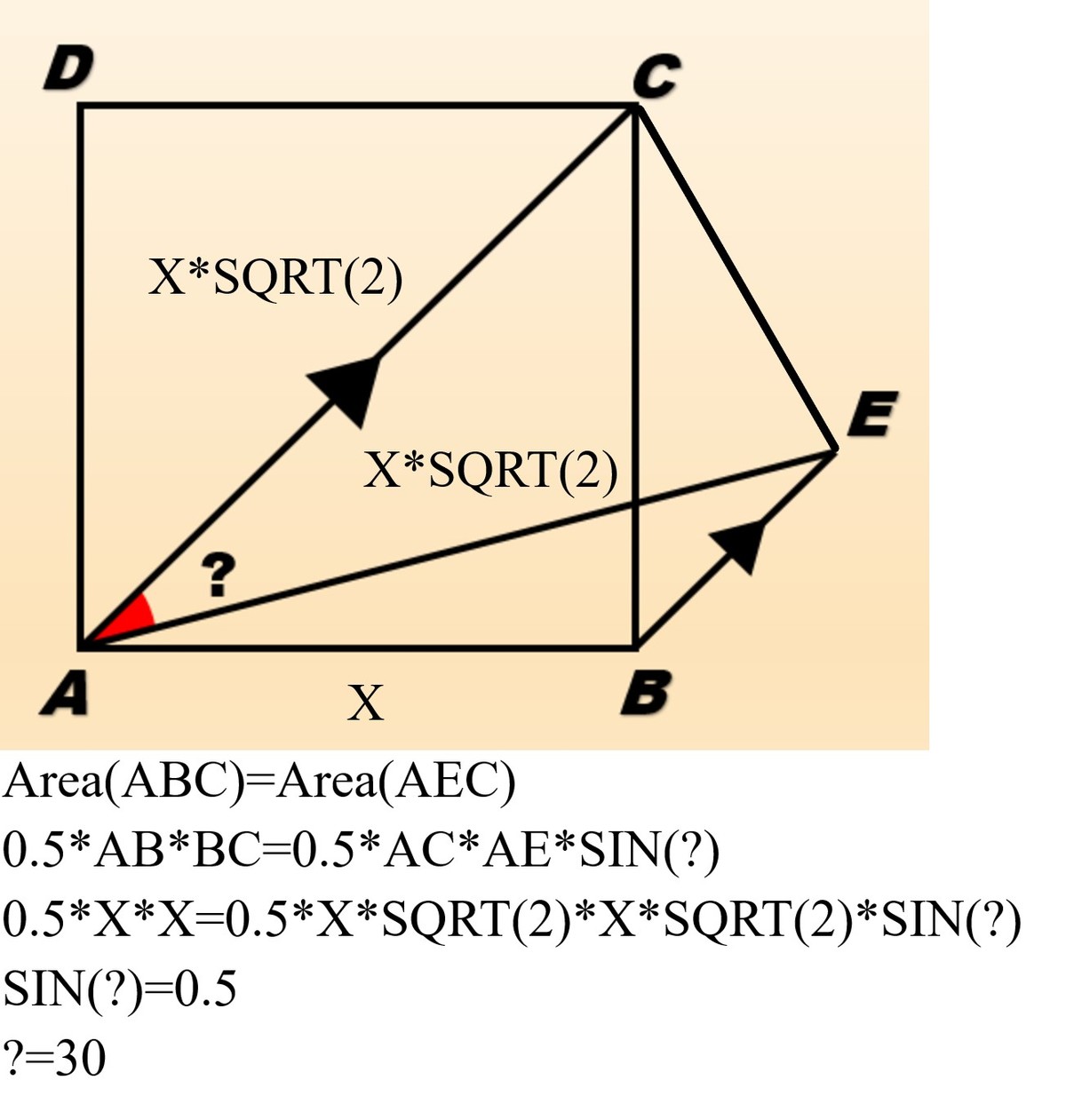
Find the angle (for beginners)

Reflect the triangle $ABE$ over the line $BE$ to get the triangle $A’BE$ .

Note that $\angle ABE = \angle A'BE =135 ^{\circ}$ . The $\angle ABA'=90 ^{\circ}$ and hence $AA'=AC=AE=A'E$ .

The triangle $AA’E$ is equilateral. Now $\angle EAA'=60^{\circ}$ . Since $\angle BAA'=45^{\circ}, \angle EAB=15^{\circ}$ , we have $\angle CAE=30^{\circ}$ .

There are different approaches and discussion in this video .

Let $|\overline {AB}|=x$ and $\angle {EAB}=α$ . Then applying sine rule to $\triangle {ABE}$ we get

$\dfrac {x\sqrt 2}{\sin 45\degree}=\dfrac {x}{\sin (45\degree-α)}$

$\implies \sin (45\degree-α)=\dfrac{1}{2}=\sin 30\degree$

$\implies \angle {CAE}=45\degree-α=\boxed {30\degree}$ .

$\angle ABE=90+45=135^\circ$

Let $AB=1$ , then $AC=AE=\sqrt{2}$

Apply sine law on $\triangle ABE$

$\dfrac{\sin \angle AEB}{1}=\dfrac{\sin \angle ABE}{\sqrt{2}}$

$\angle AEB=30^\circ$

It follows that

$\angle EAB=180-135-30=15^\circ$

Finally

$x=45-15=\boxed{30^\circ}$