Find the area of the enclosed region
If the area outside the circle and inside the circle can be expressed as , then find .
See the figure below:
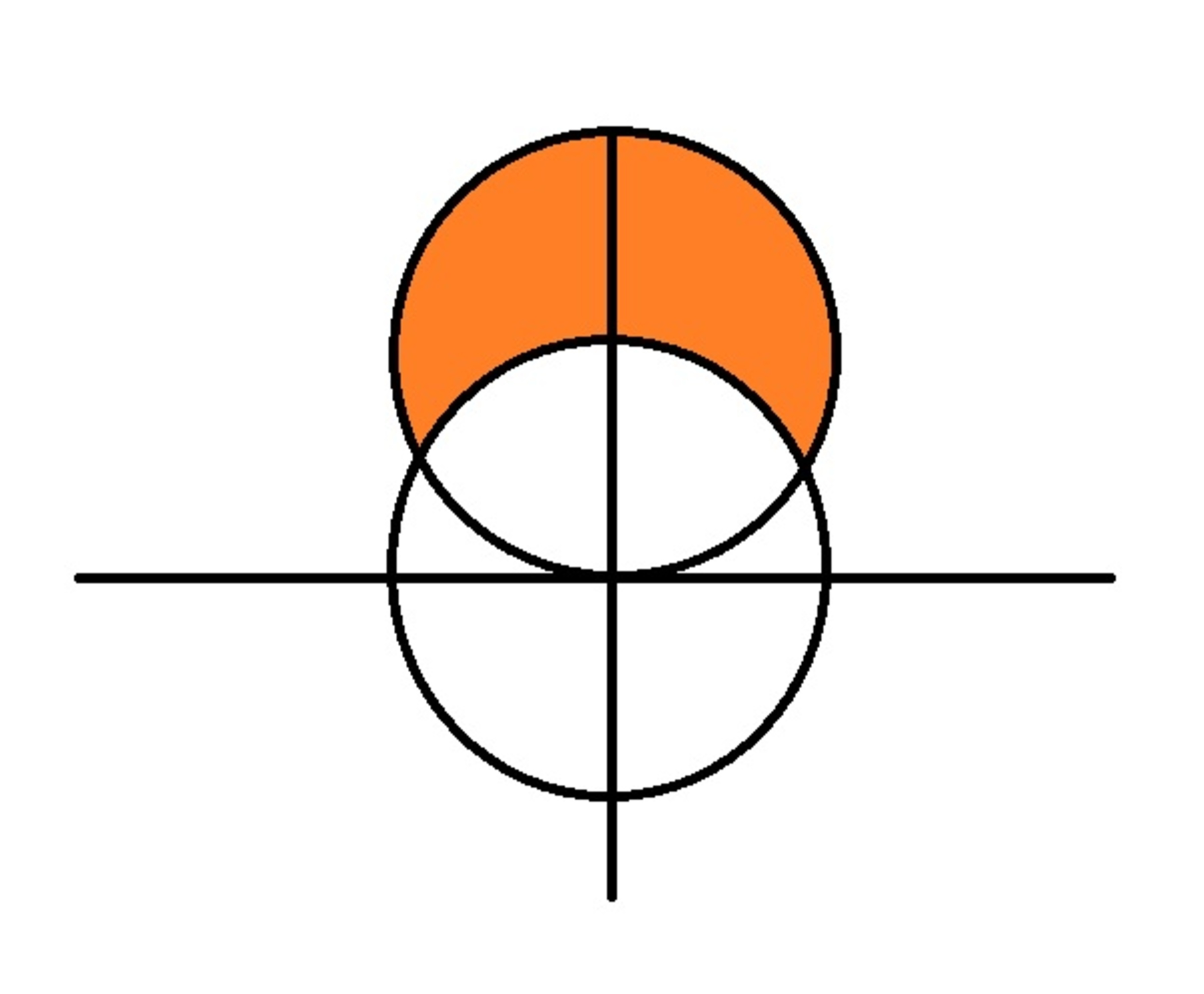
The region is described by and .
The answer is 9.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
By Calculus:
The shaded area is given by:
A = 2 1 ∫ 6 π 6 5 π ( r 2 ( θ ) − 2 2 ) d θ = 2 1 ∫ 6 π 6 5 π ( 1 6 sin 2 θ − 4 ) d θ = ∫ 6 π 6 5 π ( 4 ( 1 − cos 2 θ ) − 2 ) d θ = ∫ 6 π 6 5 π ( 2 − 4 cos 2 θ ) d θ = [ 2 θ − 2 sin 2 θ ] 6 π 6 5 π = 3 5 π − 3 π − 2 ( − 2 3 − − 2 3 ) = 3 4 π + 2 3
⟹ a + b + c = 4 + 3 + 2 = 9
By Geometry:
In rectangular coordinate system, we have:
{ x = r cos θ = 4 sin θ cos θ = 2 sin 2 θ y = r sin θ = 4 sin 2 θ = 2 − 2 cos 2 θ
⟹ x 2 + ( y − 2 ) 2 = 4 , which is a circle centered at ( 0 , 2 ) and radius 2.
We note that the segment in the overlap extends an angle of 1 2 0 ∘ with the upper circle. Therefore, the area of the shaded region
A = π R 2 − 2 ( 3 1 π R 2 − 2 × 2 1 × 2 1 × 2 3 R 2 ) = 4 π − 3 8 π + 2 3 = 3 4 π + 2 3 where R = 2
⟹ a + b + c = 4 + 3 + 2 = 9