Find the area of the shaded triangle
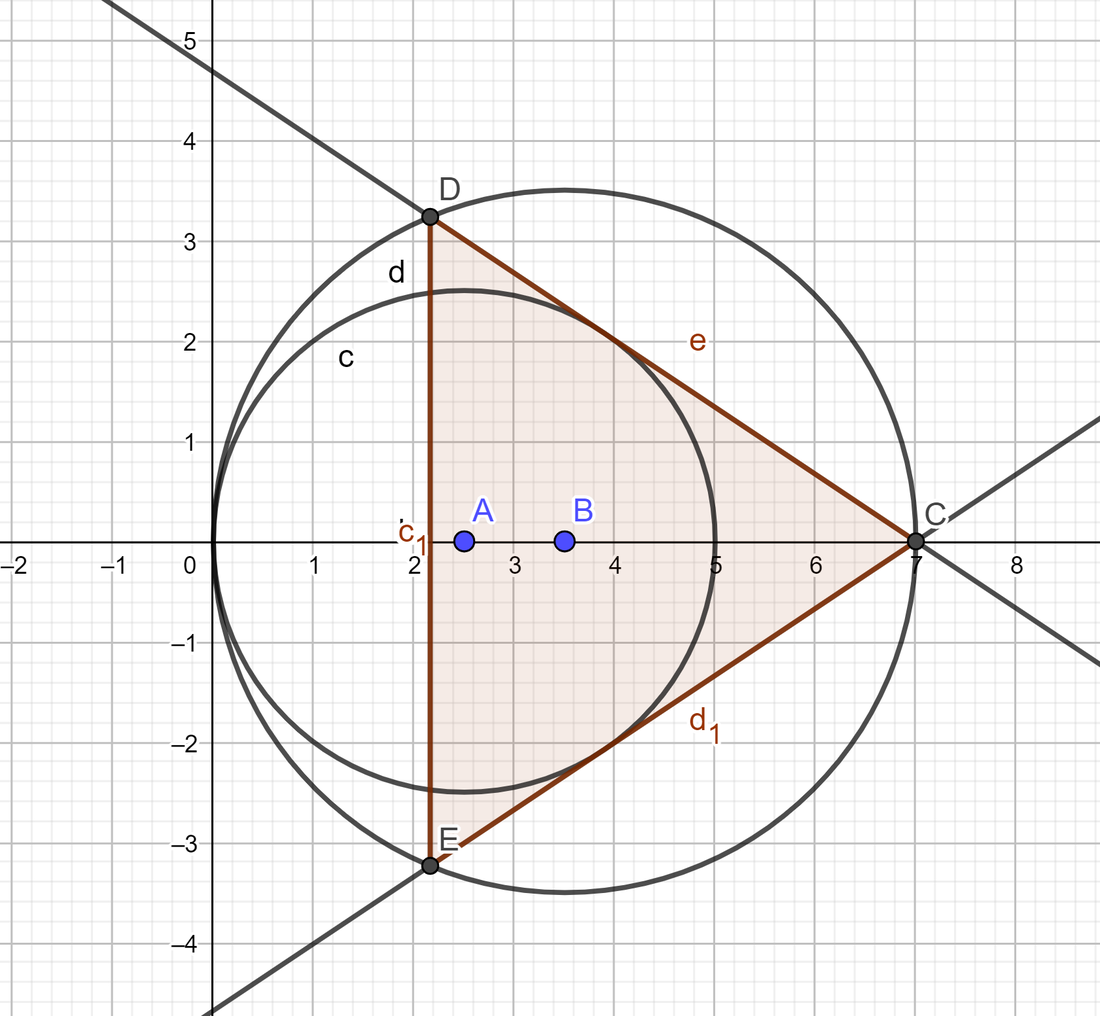
A small circle of diameter is centered at , and a bigger circle of diameter is centered at , so that the two circles are tangent to each other at the origin. From the point you draw tangents to the small circle which intersect the big circle again at points . Find the area of .
The answer is 15.65.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let O be the origin ( 0 , 0 ) , A F be perpendicular to C D , and the x -axis bisects D E at G . Since O C = 7 is a diameter of the big circle, ∠ O D C = 9 0 ∘ . Then △ O D C and △ A F C are similar. Then O C C D = A C C F ⟹ C D = A C C F × O C = A C A C 2 − A F 2 × O C = 9 1 4 1 4 .
We also note that △ G D C and △ A F C are similar. Then D G = A C C D × A F = 8 1 7 0 1 4 , C G = d f r a c C D A C × C F = 7 0 1 4 8 1 , and the area of △ C D E = D G × C G = 8 1 7 0 1 4 × 3 9 2 8 1 = 6 5 6 1 2 7 4 4 0 1 4 ≈ 1 5 . 6 .