Find the best move
Logic
Level
3
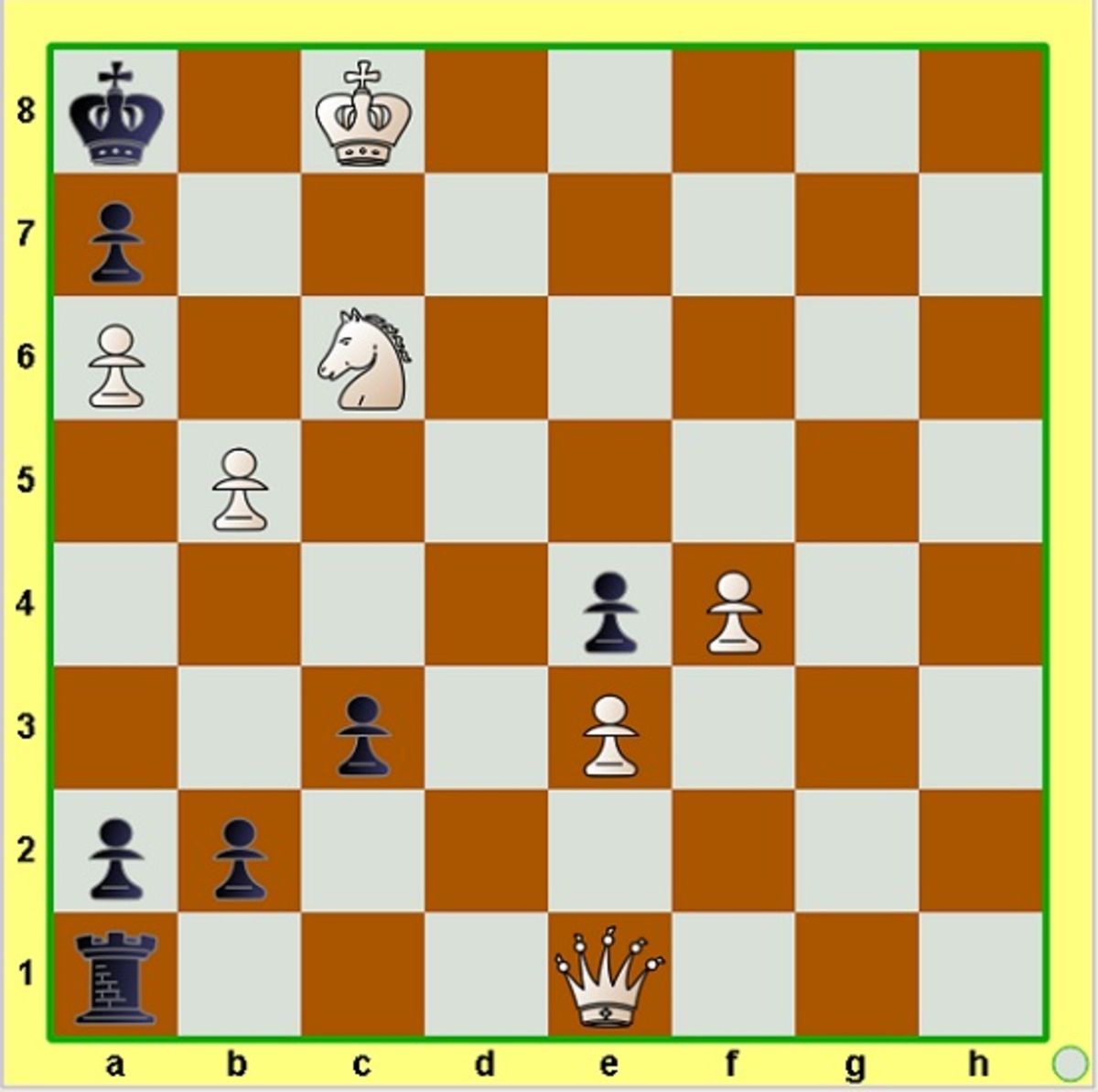It’s white turn to move. If white and black play optimally, what is the minimum number of moves for white to checkmate the black king?
Puzzle by Kraemer – 1948
The answer is 4.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
White’s main plan is to transfer the knight to c7. Solving this problem is like peeling the layers off an onion. Attempting to bring the queen into the the mortal kombat is not a good idea. For example 1. Qxc3? 2. Rg1! or 1. Qg3? 2. Rg1! or 1. Qh4? 2. Rh1! and so on. So White’s main idea is to play his knight to c7. There are three possible routes, 1. Nd8, intending Ne6-c7, 1.Ne7, intending Nd5-c7 and 1.Nb4, intending Nd5-c7.
1 Nb4! This is the first layer.
1...c2 If Black promotes with the check, then White's mating plans will be completely disrupted, so White must do something extraordinary to prevent 2...c1Q+.
2 Qc1! This spectacular move is the second layer. White simply blocks the c-pawn, preventing the check. Now it seems that Black's resources are at an end because neither 2...bxc1Q nor 2...b1Q stops White's knight tour to c7.
2...b1=B! The third layer. This imaginative defense incarcerates Black's rook and sets up a stalemate. If White had chosen one of the other first moves, his knight would now be on either d8 or e7. In neither case would it be possible to mate in two, but with the knight on b4 there is one final layer ...
3 Nd3!
3...exd3
4 Qh1#
Variations:
After 1.Nb4!, there are many variations. But it will end all in mate in 4 moves.