Find the diameter?
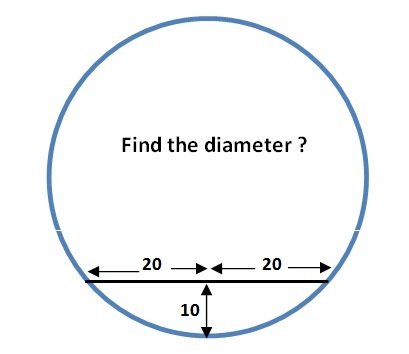
The above shows a circle. Find the diameter of this circle.
The answer is 50.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
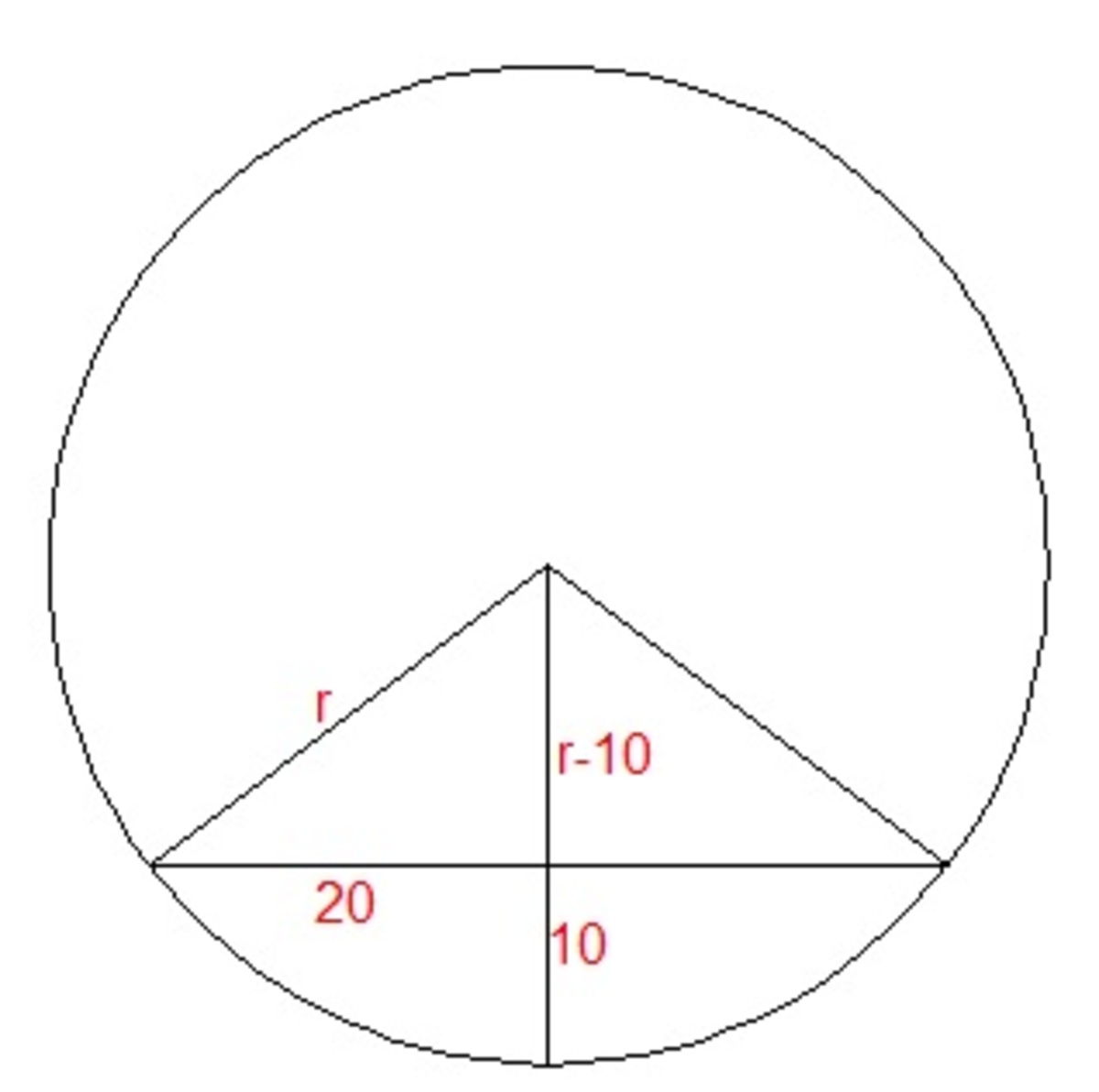 Consider the diagram on the left.
Consider the diagram on the left.
r 2 = ( r − 1 0 ) 2 + 2 0 2
r 2 = r 2 − 2 0 r + 1 0 0 + 4 0 0
2 0 r = 5 0 0
r = 2 5
d i a m e t e r = 2 r = 2 ( 2 5 ) = 5 0
r 2 − 2 0 2 = ( r − 1 0 ) 2
r 2 − 4 0 0 = r 2 − 2 0 r + 1 0 0
2 0 r = 1 0 0 + 4 0 0
2 0 r = 5 0 0
r = 2 5
Diameter = 2 5 × 2 = 5 0
Relevant wiki: Circles - Intersecting Chords
Using intersecting chord theorem, we know that the product of the two segments of the chord (20x20) is equal to the product of the two segments of the diameter (10x ?).
20x20 = 10x?
400 = 10x?
400 = 10x40
D = 10+40 = 50