Find the initial acceleration

$a\approx \frac {\mu gv_{o}} {\omega R}=1$ . We will use polar coordinates. Easy to conclude from symmetry that there is only a net force in the horizontal.Fix a particle of mass $dm=\sigma rd\theta dr$ . $f_{x}=\mu dm g\cos \alpha$ .Where $\cos \alpha =\frac {v+\omega r\sin \theta}{\sqrt {v^2+(\omega r)^2+2v\omega r\sin \theta}}$ . Approximating the denominator by neglecting the $v^2$ term and expanding binomially gives $\cos \alpha\approx \sin\theta +v_{o}\cos ^2\theta /\omega r$ The acceleration is thus obtained by $Ma_{cm}=\int_{r=0}^{R}\int_{\theta =0}^{2\pi} \mu \sigma r(\sin \theta +v_{o}\cos ^2\theta /\omega r)drd\theta=\frac{\mu gv_o}{\omega R}$

If the disk is rotating with angular velocity $ω_0$ and moving at speed $(v_0, 0)$ , the velocity at any point $(x,y)$ of the disk has velocity $(v_0-ω_0y, ω_0x)$ relative to the table.

There is a point $P=(0,h=\frac{v_0}{ω_0})$ where the movement of the disk is stationary relative to the table. Since $ω_0R>>v_0$ , P is near the centre of the disk.

All other points of the disk are moving with respect to the table, so anywhere the friction force per unit area is $\frac{dF}{dA} = \frac{μmg}{A}$ in magnitude, its direction is perpendicular to the line connecting that part of the disk and P.

By symmetry, we see that the acceleration $a_0$ must be parallel to $v_0$ , so we will add up only the x-components of the forces.

We can imagine a circle the same size as the disk, but centered at P, determining the direction of the friction forces, while the disk itself is restricting the area where these forces can work. 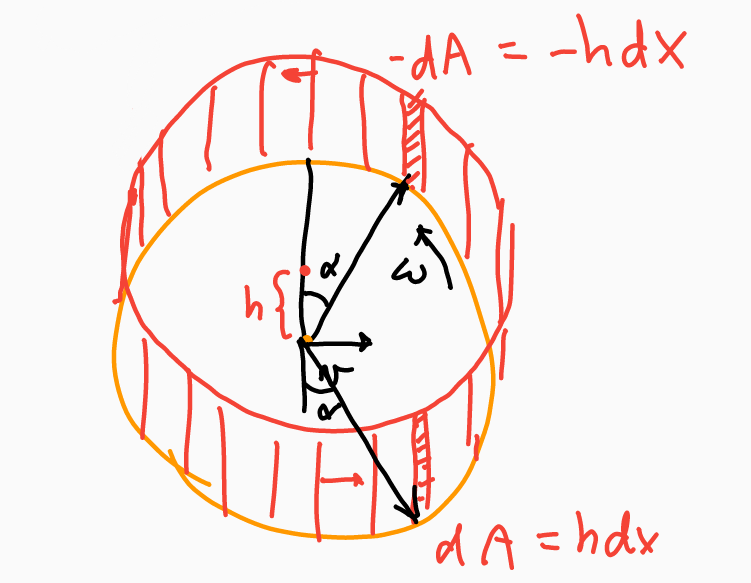For the area that the circles overlap, the force contributions cancel out. Only near the edges, there are net contributions. The excess area of the disk at the bottom edge is $dA = h dx$ At the top edge on the other hand, we are missing this same area. Because at the top edge $y>0$ the friction is in the same direction as v_0, and at the bottom edge we have exces disk area and the force is directed opposite to $v_0$ , the force gets a minus sign in both cases, when the edges add up, this results in a factor $-2$ :

The effective force contribution of a bit of area $dA$ near either of the edges then is $dF=-\frac{μmg |\cos α|}{A} dA$ where $α$ is the angle between $v_0$ and $v(x,y)$ .

When $h<<R$ , $|\cos α|$ can be approximated as $\frac{|y|}{R}$ where $\frac{|y|}{R} = \frac{\sqrt{R^2-x^2}}{R}$ .

$dF = -2\frac{μmg\cos α}{A} h dx$

$= -\frac{2μmgh}{πR^2}  \frac{\sqrt{R^2-x^2}}{R} dx$

$= -\frac{2μmgh}{πR^3} \sqrt{R^2-x^2} dx$

$F = \int_{x=-R}^{R}dF=\int_{-R}^{R} \frac{-2μmgh}{πR^3} \sqrt{R^2-x^2} dx$

$=-\frac{2μmgh}{πR^3} \int_{x=-R}^{R} \sqrt{R^2-x^2} dx$

This integral can be recognized as the area of half a disk, so

$F=-\frac{2μmgh}{πR^3} \frac{πrR^2}{2}=\frac{μmgh}{R}$

Since $μg=1$ and $h=\frac{v_0}{ω_0}$ , the initial acceleration a_0 is $a_0 =\frac{F}{m} = \frac{-v_0}{ω_0R}$

So both numbers a and b are equal to 1 and the asked expression is $1^1+1^1+1×1=\boxed{3}$ .