Find the measure of angle

All the known and deducible measures of angle are shown in the figure. Let $\angle ADC = \theta$ . Then by sine rule, we have

$\begin{cases} \dfrac {AC}{CD} = \dfrac {\sin \angle ADC}{\sin \angle CAD} \\ \dfrac {BC}{CD} = \dfrac {\sin \angle ADB}{\sin \angle CBD} \end{cases}$ .

Since $AC=BC$ , we have:

$\begin{aligned} \frac {\sin \angle ADC}{\sin \angle CAD} & = \frac {\sin \angle ADB}{\sin \angle CBD} \\ \frac {\sin \theta}{\color{#3D99F6}\sin 24^\circ} & = \frac {\color{#D61F06}\sin (228^\circ - \theta)}{\sin 12^\circ} & \small \color{#3D99F6} \text{Note that }\sin (2x) = 2\sin x \cos x \\ \frac {\sin \theta}{\color{#3D99F6}2} & = \color{#3D99F6}\cos 12^\circ \color{#D61F06} \sin (\theta - 48^\circ) & \small \color{#D61F06} \text{Since }\sin (180^\circ - x) = \sin x \\ {\color{#3D99F6}\sin 30^\circ} \sin \theta & = {\sin 78^\circ} \sin (\theta - 48^\circ) & \small \color{#3D99F6} \text{Assuming } \theta - 48^\circ = 30^\circ \implies \theta = 78^\circ \\ \sin \theta & = \sin 78^\circ & \small \color{#3D99F6} \text{proving that the assumption is correct.} \\ \implies \theta & = 78^\circ \end{aligned}$

Therefore, $\angle ACD = 180^\circ - \angle CAD - \angle ADC = 180^\circ - 24^\circ - 78^\circ = \boxed{78}^\circ$ .

Aly, feel free to take this image and add it to your problem.

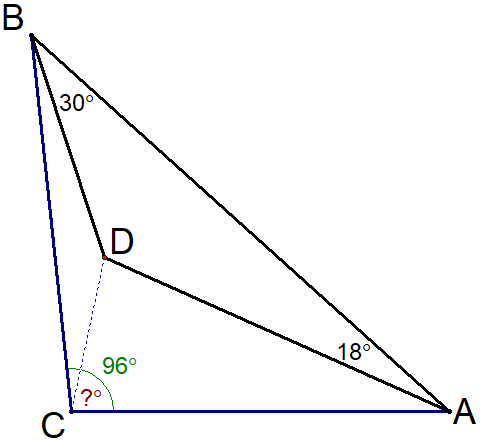
Since $\triangle ABC$ is isosceles $\angle ABC=\angle BAC=42$ and we can easily derive $\angle DAC=24$ and $\angle ADB=132$

For simplicity let $AC=BC=1$ then by the law of sines on $\triangle ACB$ , $AB=\frac{\sin{96}}{\sin{42}}=\frac{\sin{84}}{\sin{42}}=\frac{2\sin{42}\cos{42}}{\sin{42}}=2\cos{42}=2\sin{48}$ .

Now using the law of sines on $\triangle ADB$ , $\frac{AD}{\sin{30}}=\frac{2\sin{48}}{\sin{132}}$ , $AD=\frac{\sin48}{\sin{132}}=\frac{\sin{48}}{\sin{48}}=1$

So $\triangle ADC$ is isosceles. $\angle DAC=42-18=24$ and $\angle ACD = \frac{180-24}{2}=\boxed{78}$