$\angle C = ?$

By Thales theorem angle ADB is 90°. And because D is midpoint of line segment AC we can say that triangle ACB is isosceles. Now because angle ACH is congruent to angle CAB and triangle ACB is isosceles we can say that angles CAB and BCA are congruent too, thus line CA is angle bisector of angle BCH. Let E be the foot of perpendicular from B to line CH and let $r$ be the radius of the circle. We now know that $|BE|=r$ and $|CB|=|BA|=2r$ , from this we can easily calculate that the angle BCE is 30° and thus $|ACH|=\frac{1}{2}|BCH|=15°$ .

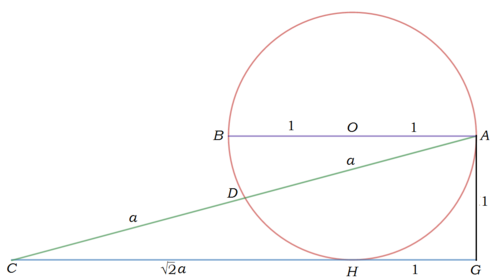
Let the radius of circle $O$ be $1$ and $AD=CD=a$ . Then the height of $\triangle ACG$ , $AG=1$ . By tangent-secant theorem , we have $CH^2 = CA \cdot CD = 2a^2 \implies CH=\sqrt 2a$ . By Pythagorean theorem ,

$\begin{aligned} AG^2 + CG^2 & = AC^2 \\ 1^2 + (1+\sqrt 2a)^2 & = (2a)^2 \\ 1 + 1 +2\sqrt 2 a + 2a^2 & = 4a^2 \\ a^2 - \sqrt 2a - 1 & = 0 \\ \implies a & = \frac {\sqrt 2 + \sqrt 6}2 & \small \blue{\text{Since }a > 0} \\ \sin \angle ACH & = \frac {AG}{AC} = \frac 1{\sqrt 2 + \sqrt 6} \\ \implies \angle ACH & = \boxed{15}^\circ \end{aligned}$

Let the radius of the circle be $r$ and magnitude of $\angle {ACH}$ be $α$ . Then, since $\angle {BDA}$ , being a semicircular angle, is $90\degree$ , $|\overline {AD}|=2r\cos α\implies |\overline {AC}|=4r\cos α$ . Applying sine rule to the $\triangle {ACH}$ we get $\dfrac{4r\cos α}{\sin 135\degree}=\dfrac{r\sqrt 2}{\sin α}\implies \sin 2α=\dfrac{1}{2}\implies 2α=30\degree\implies α=\boxed {15\degree}$ .