Finddd
Level
pending
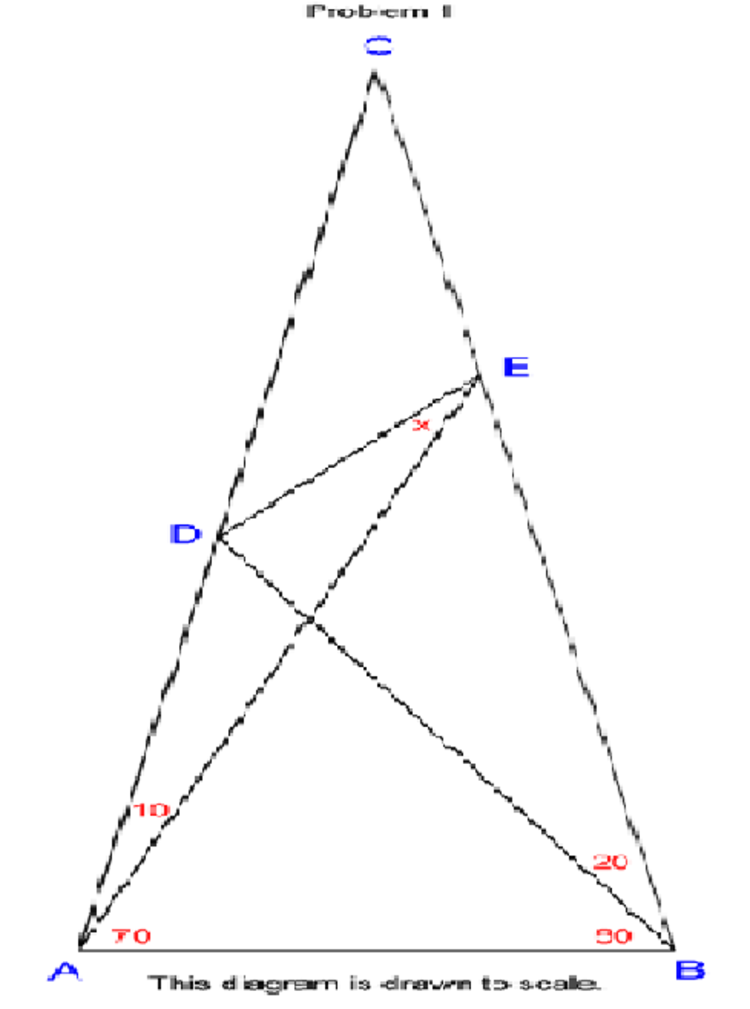 Determine the value of X.
Determine the value of X.
The answer is 20.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Calculate some known angles: ACB = 180-(10+70)-(60+20) = 20° AEB = 180-70-(60+20) = 30° Draw a line from point D parallel to AB, labeling the intersection with BC as a new point F and conclude: DCF ACB CFD = CBA = 60+20 = 80° DFB = 180-80 = 100° CDF = CAB = 70+10 = 80° ADF = 180-80 = 100° BDF = 180-100-20 = 60° Draw a line FA labeling the intersection with DB as a new point G and conclude: ADF BFD AFD = BDF = 60° DGF = 180-60-60 = 60° = AGB GAB = 180-60-60 = 60° DFG (with all angles 60°) is equilateral AGB (with all angles 60°) is equilateral CFA with two 20° angles is isosceles, so FC = FA
Draw a line CG, which bisects ACB and conclude: ACG CAE FC-CE = FA-AG = FE = FG FG = FD, so FE = FD
With two equal sides, DFE is isosceles and conclude: DEF = 30+x = (180-80)/2 = 50 Answer: x = 20°