Finding the Sum?

Here, $\begin{aligned} 3 + 5 +\cdots + 101 & = 3 + 5 + \cdots+ \,({\color{#3D99F6}1}+100) \\& = \underbrace{{\color{#3D99F6}1}+3+5+\cdots + 99 }_{\text{Given}} + 100 \\& = 2500 +100 =2600\end{aligned}$

Alternatively: Given that , $1+\underbrace{ 3 +5 +\cdots+ 99 }_{\text{S}}=2500\implies\underbrace{ 3 +5 +\cdots+ 99 }_{\text{S}}= 2499$ We need to find $\underbrace{3 + 5+7+\cdots +99 }_{\text{ S}} + 101 = 2499 +101 =\boxed{2600}$

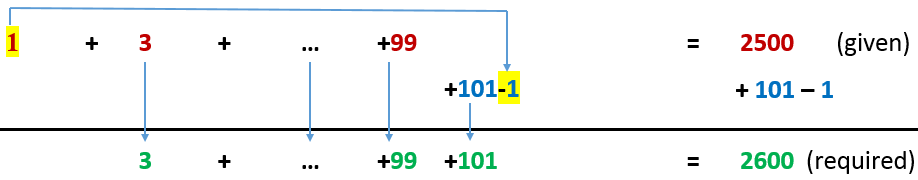
Given, is the sum of all odd numbers from $1 - 99$ : $1 + 3 + 5 + 7 + ... + 99 = 2500$

Now we are asked to find the sum of all odd numbers from $3 - 101$ . It is simple. Add $101$ and subtract $1$ on both sides of the equation : $\begin{aligned} \cancel{1} + 3 + 5 + 7 + ... + 99 + 101 - \cancel{1} = 2500 + 101 - 1 \\ \implies \color{#3D99F6} 3 + 5 + 7 + ... + 99 + 101 = 2600 \quad \quad \quad \quad \quad \\ \end{aligned}$

In the know series 1 + 3 + 5 + … + 99 = 2500, excluding “1” and including “101” gives 3 + 5 + …+ 101 = 2500 - 1 + 101 = 2500 - 1 + 100 + 1= 2500 + 100 =2600.

Relevant wiki: Arithmetic Progression Sum

$3,5,7,...,101$ is an Arithmetic Progression with a common difference of $2$

We need to find the number of terms by using the formula $a_n=a_1+(n-1)d$ . Substituting, we have

$101=3+(n-1)2$

$98=(n-1)2$

$\dfrac{98}{2}=n-1$

$n=50$

Now, we will find the sum of the terms by using the formula $s=\dfrac{n}{2}(a_1+a_n)$ . Substituting, we have

$s=\dfrac{50}{2}(3+101)=\boxed{2600}$

$3+5+7+...+101=(1+2)+(3+2)+(5+2)+...+(99+2)=(1+3+5+...+99)+50\times 2=2500+100=\boxed{2600}$

To go from $1 + 3 + 5 + \cdots + 99 = 2500,$ to $3 + 5 + 7 + \cdots + 101,$ we must subtract $1$ and add $101$ . This evens out to a $+ 100.$

Since $2500 + 100 = 2600, 3 + 5 + 7 + \cdots + 101 = 2600$ as well.

That was simple. You have to just subtract 1 and add 101.

Let's call $f(x)=2x+1$ positive odd number function.

In one of the Brilliant's solutions,they said that:

$f(0)+f(1)+f(2)+\dots+f(\frac{n-1}{2})=(\frac{n+1}{2})^2$

Let's $b={3+5+7+..+101}$

Then, $b$ equals:

$b=(\frac{101+1}{2})^2-f(0)=(\frac{102}{2})^2-1=51^2-1=2601-1=\boxed{\large{2600}}$

Odd number series from 1 till 99 have the following sum: $1 + 3 + 5 + \ldots + 99 = 2500$

The next series are literally the same shifting one step to the right. Starts from 3 and finishes with 101. $Thus:\ 2500 - 1 + 101 = 2600$

Simple solution:2500-1+101=2600.

If we implement this task in some programming language like Python, then it would look like this in the picture below.

Substract the first sum from the second, so you get

101 + 99 - 99 +... + 3 - 3 - 1 = 101 - 1 = 100. The second sum is 100 more, so 2500 + 100 = 2600.

The formula to find the sum of an arithmetic sequence: $sum =\dfrac{n}{2}(a_1+a_n)$ To find n (number of terms): $n=\frac{ a_n-a_1 }{difference}+1$ Sequence given: $3 , 5 , 7 , \cdots , 101$ We first need to find n :
$n=\frac{101-3}{2}+1$ $n=49+1$ $n=50$ Now, we can find the sum: $Sum=\frac{50}{2} \times (3+101)$ $=25\times104$ $=\boxed{2600}$

The first sum may be written in sigma notation as $\displaystyle\sum_{k=1}^{50} (2k-1) = 2500$
The second is written as $\displaystyle\sum_{k=1}^{50} (2k+1)$ which is equivalent to $\displaystyle\sum_{k=1}^{50} (2k-1) + \displaystyle\sum_{k=1}^{50} 2$
$\displaystyle\sum_{k=1}^{50} (2k-1) + \displaystyle\sum_{k=1}^{50} 2$ can then be simplified to $2500+ 2 \displaystyle\sum_{k=1}^{50} 1 = 2500+100 = 2600$

i got it right but they wrote it pretty confusing, i didnt realized at the beginning if the 101 is after the 99 or not.

You add the last number with the first one, and so on:

(3+101) + (5+99) + (7+97) + ... + (101+3)
= 104 + 104 + 104 + ... + 104

The amount of 104's of this last sum is 50, so we multiply 104*50 and divide it by 2 because we have twice the sum.

Therefore: 104*(50/2) = 2600

My suggestion is to write up both sequences up on top of each other like this:

$\thinspace1+3+5+7+...+99\quad\quad\thinspace =2500$

$\quad\quad3+5+7+...+99+101 = ?$

That way, it is easy to see that the series below lacks a 1 and has one more 101. The difference is therefore +100 and the total sum is 2600.

$\begin{aligned} 1+3+5+7 + \cdots + 99 & = 2500 \\ 1+3+5+ 7 + \cdots + 99 \color{#D61F06} -1 & = 2500 \color{#D61F06} - 1 \\ 3+5+7+\cdots + 99 \color{#3D99F6} +101 & = 2499 \color{#3D99F6} + 101 \\ 3+5+7 + \cdots + 101 & = \boxed{2600} \end{aligned}$

The two series are the same except the top series has 1 in front, which the second is without, and the second has 101 added at the back instead.

So the sum of the second is up by $101-1=100$ , so it is equal to $2500+100=\boxed{2600}$

$\begin{aligned} &\quad\space3+5+7+\cdots+101\\ &=1+3+5+\cdots+99-1+101\\ &=2500+100\\ &=\boxed{2600} \end{aligned}$

There is a great trick which I used to solve this problem.

First I choose a limit for ex- I want to add the consecutive odd numbers from 1to 9

So I add 1 to 9 ( 9 + 1 ) = 10.

So, now I divide it by 2 ( 10/2 ) = 5

and I square the number. ( 5 * 5 ) = 25

Now, In the given question.

I need to go from 3 to 101. Now, here I used my brain. I considered that I want to go from 1 to 101 and applied all the steps.

==> Add -> 101 + 1 = 102

==> Divide -> 102 / 2 = 51

==> Square the number = 51 * 51 = 2601 ( It's easy just multiply or else I have tricks)

Now, 2601 isn't my answer as I started from 1, but now its easy for me that I will just subtract 1 from it.

= 2601 - 1 = 2600

Here is your answer in a clever way.

Let us consider 3+5+7......+101 an A.P. Solving for nth term we get 101=50th term. Next we solve for S50 that is the sum of first 50 terms of the A.P.We get 2600.

From (my) theorem:

$\displaystyle \sum_{n=1}^{k} an+b = \frac {ak(k+1)}{2}+bk$

We can replace the variables and find the required sum. First, we can see that $2n+1 = 3, 5, 7...$ , which is the series we are using in this case. So $a=2$ and $b=1$ . Then we find $k$ in $2k+1=101$ , which is 50. So $3+5+7... + 101 = \frac{(2)(50)(50+1)}{2} + (1)(50) = 2600.$

Link for the demonstration of the theorem (only spanish, sorry): https://www.scribd.com/document/384794060/Suma-de-Toda-Funcion-Lineal

2500 - 1 +101 = 2600

1+3+...+99=2500 Add +1 and -1 to 3+5+...+101=(1+3+...+99)+101+(-1)=2500+100=2600

According to the legend, genius Gauss solved a similar (and more complicated) problem with this method as a very young child:

Expanding the sequence a bit more you'll have that

$1+3+5+\cdots+95+97+99=2500$

Then all you have to do is add up the first integer with the last integer of the sequence, the second with the one preceding the last, etc.:

$(1+99) + (3+97) + (95+5) + \cdots = (100)+(100)+(100)+\cdots$

Given that the interval $[1..99]$ contains 100 integers only 50 pairs can be made, but since our first sequence only contains odd numbers (which is half of all integers in $[1..99]$ ) only 25 pairs can be made:

$25 (100) = 2500$

$2500 = 2500$

Now using this method on the other sequence implies that:

$(3+101)+(5+99)+(7+97)+\cdots=(104)+(104)+(104)+\cdots$

$25(104)=\boxed{2600}$

So the algorithm goes 1+3+5+7+...+99=2500. That means every count it adds 2. Now you have to know how many counts you have got. In this case 50. Know the algorithm goes 3+5+7+9+...+101=?. The things you know it needed 50 counts to get to ?. And you know every count is +2. So you do: 50 counts 2 because you have got +2 at every count means: 50 2=100+2500=2600 That was the way i got the answer and I hope its helpful. ;D

Dumbest question ever! I don't want to post a solution

2500-1+101=2600

You're taking away 1 and adding 101. So the entire equation has gone up in value by 100.

The easiest way is to pair off the numbers 101 plus 3 is 104 There are 25 pairs each adding to 104 104 times 25 = 2600

Given sum of 1st series is 2500 but after calculating sum from AP formula we have the sum as 4950. Similarly , calculating the sum of 2nd series from AP formula we have the sum as 5148 but the required sum must be in the same ratio as the ratio is obtained from the sums of the 1st series. So we have the required sum as, (2500/4950)×5148 = 2600

1+2=3, 3+2=5, 5+2=7....99+2=101

Apparently, 2 is the difference for each complementary entry. So, count the number of times you'll need to add 2 between 1 and 99 (which is fifty times, since there are fifty odd numbers between 1 and 99), multiply it by 2 (2x50=100) and add it to 2500 (=2600).

The numbers can be divided into groups. Take the sum of the first number and the last one that is 100 in the 1..99 case and 104 in the 3..101 case. Imagine keep "collapsing" the sequence by taking again the first and the last number 3 and 97 and 5 and 99 respectively getting 100 and 104 again. In both case we have 50 numbers resulting in 25 groups. As a result in the first case we get 25 * 100 = 2500 and in the second 25 * 104 = 2600.