Flux got a bit Complicated

Electricity and Magnetism Level 1

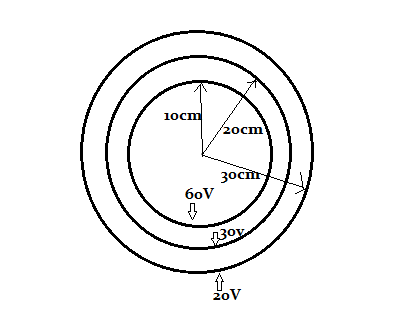
Find the total flux of the electric field through a square of edge 40 cm 40\text{ cm} placed tangent to the 30 V 30 \text{ V} equi-potential sphere.

Assume that the charge density, if any, outside the 60 V 60 \text{ V} equi- potential surface is uniform.

2 π 2\pi 8 π 8\pi 4 π 4\pi 12 π 12\pi 16 π 16\pi

Soumyadip Bijuli by Soumyadip Bijuli , 22, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Kq/r = V , q/4πEr= V,
q/E = V4πr , q/6E= V4πr/6. Put the values answer is 4π Vm

12 Helpful 6 Interesting 7 Brilliant 25 Confused

Why is the final flux one-sixth

Shefali Srivastava - 2 years ago

Log in to reply

Cuz 1 square can only cover 1/6 part of a sphere. Imagine it as the face of cube enclosing the sphere.

Ayush Anand - 2 years ago

it is divided by 6, because in the question it is given that the centre of square touches that same 30V equipotential surface, visualize in 3d that there can be 6 more like squares like this one, to form a cube, and by symmetry, it is 1/6th

Aryan Kumar - 1 year, 11 months ago

1 pending report

Vote up reports you agree with

×

Problem Loading...

Note Loading...

Set Loading...