for a good day of rest

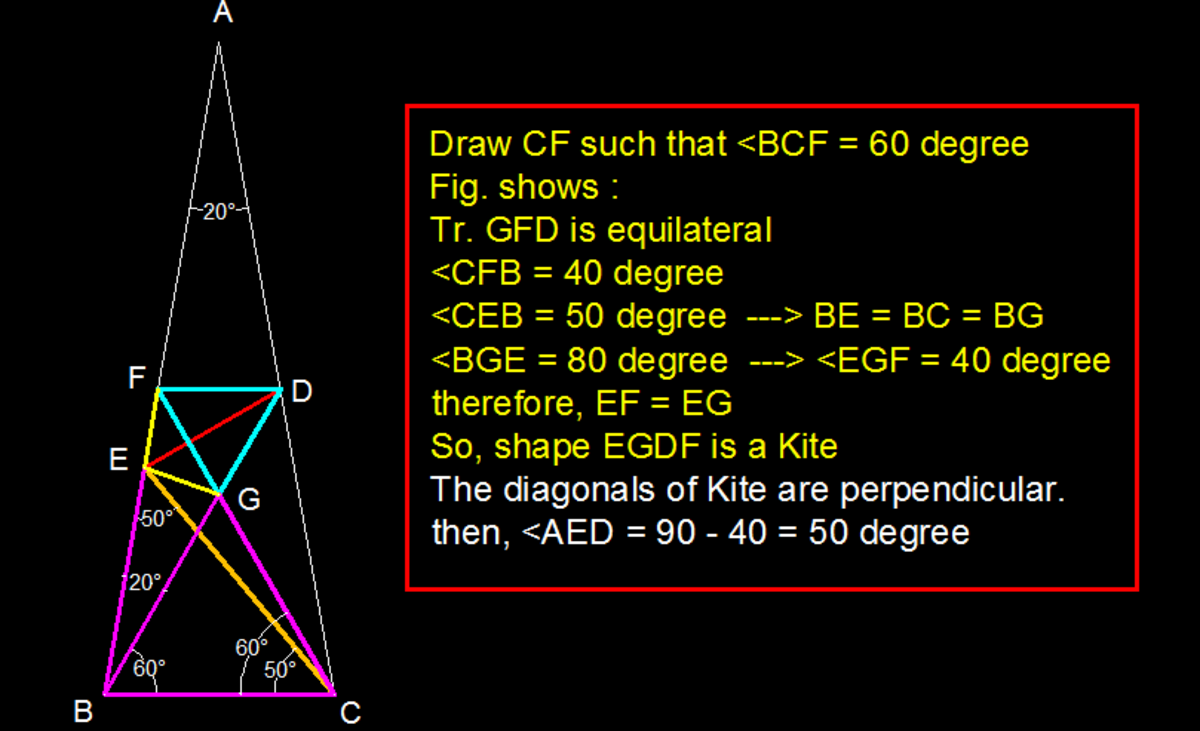
This is the famous problem Langley's Adventitious Angles where angle BDE=30. From there it is easy to get the answer.
Several solutions can be had from Google.com. Hence I am not putting them here. One such is given below.
link text

I would like to share a hint for this problem make a construction that one triangle can be made equilateral rest is solved

• Assume that: BC=1, we can simply prove that BE=BC=1, BDC angle = 40 degrees.
• We can easily calculate: AB=AC=1/(2sin(10degrees)), then AE=1/(2sin(10degrees))-1.
• Using BD/sin(BCD angle)=BC/sin(BDC angle) we've got: BD=2cos(40degrees).
• Then, we prove that: AC/AE=1/(1-2sin(10degrees))=2cos40degrees=BD/BE that means we have to prove: 1=2cos(40) (1-2sin(10)). This is similar to 1=2sin(50)-2(sin(50)-sin(30). (this is true).
• Then we have BD/AC=BE/AE and ABD angle=20=EAC angle. This leads to: BED triangle is similar to AEC triangle, then we have BDE angle=ACE angle=30 degrees and x=BDC angle + ABD angle=50 degrees.

we draw a "trisect" the angle DBC, forming three angles of 20 degrees. The tip is, therefore, find isosceles triangles and an equilateral triangle. Well, that's it, sorry that I can not draw .... thanks