Areas and Special Lines
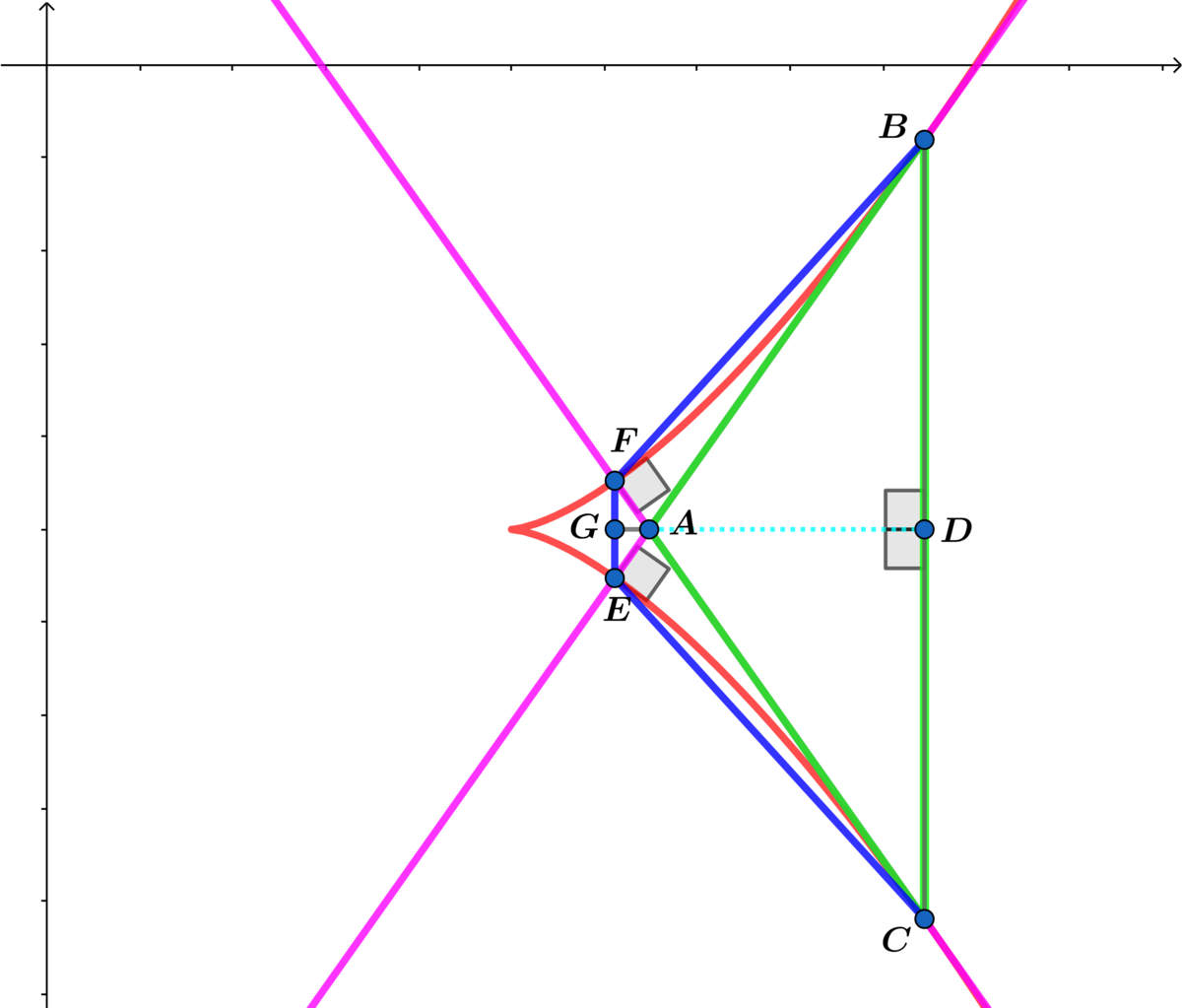
There are two lines which are both tangent and normal to the curve .
The two tangents intersect at and one of the tangents is tangent to the curve at and is normal to the curve at and the other tangent is tangent to the curve at and is normal to the curve at .
Let be the area of the trapezoid and be the area of and .
If can be expressed as , where and are coprime positive integers, find .
The answer is 797.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let y = t 3 − 1 ⟹ x = t 2 + 1 ⟹ d x d y ∣ ( t = t 1 ) = 2 3 t 1 ⟹ the tangent line to the curve at ( x ( t 1 ) , y ( t 1 ) ) is: y − ( t 1 3 − 1 ) = 2 3 t 1 ( x − ( t 1 2 + 1 ) )
Let the line be normal to the curve at ( x ( t 2 ) , y ( t 2 ) ) ⟹ ( t 2 − t 1 ) ( t 2 2 + t 1 t 2 + t 1 2 ) = 2 3 t 1 ( t 2 − t 1 ) ( t 2 + t 1 ) ⟹ 2 1 ( t 2 − t 1 ) ( 2 t 2 2 − t 1 t 2 − t 1 2 ) = 0 t 1 = t 2 ⟹ t 2 = − 2 t 1
Since the tangent is also normal to the curve at ( x ( t 2 ) , y ( t 2 ) ) ⟹ 4 9 t 1 t 2 = − 1 ⟹ 8 9 t 1 2 = 1 ⟹ t 1 = ± 3 2 2 ⟹ the two slopes are ± 2 .
slope = 2 and t 1 = 3 2 2 ⟹ y 0 − 2 x 0 = 2 7 − 3 5 2 − 2 7
slope = − 2 and t 2 = 3 2 ⟹ y 0 + 2 x 0 = 2 7 3 5 2 − 2 7
⟹ y 0 = − 1 and x 0 = 2 7 3 5 and the two tangents intersect at A : ( 2 7 3 5 , − 1 ) .
B : ( 9 1 7 , 2 7 1 6 2 − 2 7 )
C : ( 9 1 7 , , 2 7 − 1 6 2 − 2 7 )
D : ( 9 1 7 , − 1 )
A D = 2 7 1 6 and B C = 2 7 3 2 2
⟹ the area A 2 of △ A B C is A 2 = 2 1 ( B C ) ( A D ) = 7 2 9 2 5 6 2 .
F : ( 9 1 1 , 2 7 2 2 − 2 7 )
E : ( 9 1 1 , 2 7 − 2 2 − 2 7 )
The midpoint of E F is G : ( 9 1 1 , − 1 )
G D = 3 2 and F E = 2 7 4 2
⟹ the area A 1 of trapezoid C E F B is A 1 = 2 1 G D ( F E + B C ) = 9 4 2
⟹ A = A 1 − A 2 = 7 2 9 6 8 2 = β α 2 ⟹ α + β = 7 9 7 .