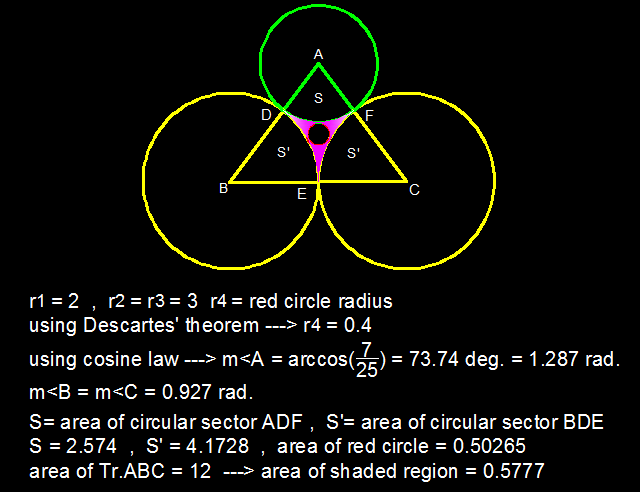
Four Circles

Let us draw a triangle formed by the centers of the circles of radius 3 and the center of the circle of radius 2 that can be denoted as $A, B$ and $C,$ respectively. Look at the figure below. Let us introduce a rectangular coordinate center such that the point $A$ coincides with the origin and the point $B$ coincides with $(6, 0).$ Since $|AC|=3+2=5,$ and the point $C$ has coordinates $(3,b),$ then $\sqrt{3^2+b^2}=5^2,$ so $b=4.$ Let us denote the radius of the smallest of the circles by $x$ and the coordinates of its center $D$ by $(3, y).$ Then $|AD|-3=\sqrt{3^2+y^2}-3=x$ and $|CD|-2= (4-y)-2=x.$ Solving the system formed by these two equations, we get that $y=\frac{8}{5}$ and $x=\frac{2}{5}.$

Besides that, it easy to see that $\sin A=\sin B = \frac{4}{5},$ or, equivalently, the angles of the triangle in the figure are $A=\sin^{-1}{\frac{4}{5}}, B=\sin^{-1}{\frac{4}{5}}$ and $C=\pi -2\sin^{-1}{\frac{4}{5}}.$

Now we can use the fact that the shaded area $K$ is equal to the area of the triangle minus the sum of the areas of the circular sectors at the corners and the area of the small circle. So $K= \frac{1}{2}*5*6 *\sin A- \frac{1}{2}*3^2 *\sin^{-1}\frac{4}{5}-\frac{1}{2}*3^2 *\sin^{-1}\frac{4}{5}-\frac{1}{2}*2^2 *(\pi -2\sin^{-1}{\frac{4}{5}})- (\frac{2}{5})^2 \pi\approx \boxed{0.58}.$