Four Equilateral triangles

Using angle-chasing, it can be shown that the 3 blue triangles are similar. In each of these similar blue triangles, the opposite side of the 60 degree is of the same length, then these 3 blue triangles are congruent.

Next, let $a$ and $b$ be the side length of the triangles with areas 20 and 45 respectively. Then $a^2:b^2=20:45=4:9$ , which implies that $a:b=2:3$ . Let $a=2k$ and $b=3k$ . Let $c$ be the side length of the yellow triangle.

As the 3 blue triangles are congruent, each of the triangles has side $2k, 3k, c$ and an angle 60 degree (as shown).

Using Cosine Rule, $c^2=a^2+b^2-2ab \cos 60 ^\circ = 7k^2$ .

Now $a^2:b^2:c^2=4:9:7=20:45:35$ . So the area of the yellow triangle is 35.

Watch this video for the explanation.

Let the length of each side of orange $\triangle {}$ be $a$ , red $\triangle {}$ be $b$ and of the yellow $\triangle {}$ be $c$ . Then $a^2=\dfrac{80}{\sqrt 3}, b^2=\dfrac{180}{\sqrt 3}$ , and $c^2=a^2+b^2-ab$ . So the area of the yellow $\triangle {}$ is $20+45-\dfrac{\sqrt {3\times {80}\times {180}}}{4\sqrt 3}=65-30=\boxed {35}$

The three blue triangles all have angles that are $60°$ , $\theta$ , and $120° - \theta$ and one side congruent to the side of the yellow equilateral triangle, so they are all congruent by the ASA congruency theorem.

Let $A$ and $a$ be the area and side of the left equilateral triangle, $B$ and $b$ be the area and side of the right equilateral triangle, $C$ be the area of the yellow equilateral triangle, $D$ be the area of each of the blue triangles, and $E$ be the area of the large equilateral triangle. The sides of $E$ are then $a + b$ , and $E = C + 3D$ .

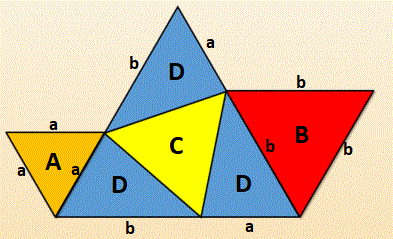
By triangle area equations,

$A = \frac{\sqrt{3}}{4}a^2$

$B = \frac{\sqrt{3}}{4}b^2$

$D = \frac{1}{2}ab \sin 60° = \frac{\sqrt{3}}{4}ab = \sqrt{AB}$

$E = \frac{\sqrt{3}}{4}(a + b)^2 = \frac{\sqrt{3}}{4}a^2 + 2\frac{\sqrt{3}}{4}ab + \frac{\sqrt{3}}{4}b^2 = A + 2D + B = A + B + 2\sqrt{AB}$

$C = E - 3D = (A + B + 2\sqrt{AB}) - 3\sqrt{AB} = A + B - \sqrt{AB}$

In this question, $A = 20$ and $B = 45$ , so $C = 20 + 45 - \sqrt{20 \cdot 45} = \boxed{35}$ .