Four in Five
Geometry
Level
3
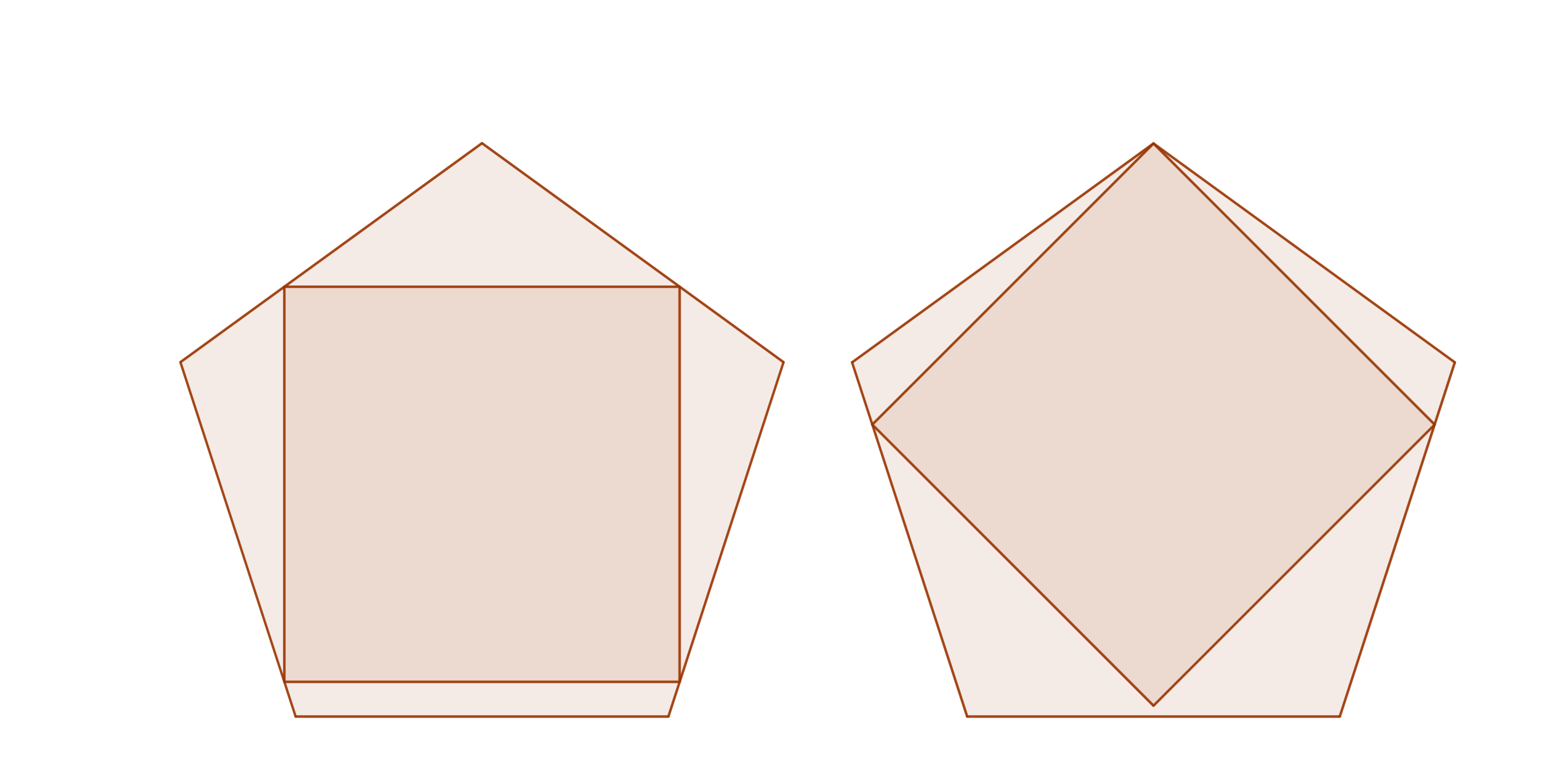
The two regular pentagons are congruent. Which inscribed square is larger?
The left square
The right square
They are both equal
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Label the diagram as follows:
Let the side of each pentagon be 1 , let the side of the left square be s 1 , and let the side of the right square be s 2 . Also, let A C = x , so that C D = 1 − x .
Since the interior angle of a regular pentagon is 1 0 8 ° , ∠ C D E = ∠ F G H = 1 0 8 ° and ∠ B A C = 2 1 0 8 ° = 5 4 ° , which by corresponding angles means that ∠ D C E = ∠ B A C = 5 4 ° and ∠ C E D = 1 8 0 ° − ∠ E C D − ∠ E D C = 1 8 ° .
Then from right △ A B C , sin 5 4 ° = 2 x s 1 , and from the law of sines on △ C D E , sin 1 0 8 ° s 1 = sin 1 8 ° 1 − x , and these two equations solve to s 1 ≈ 1 . 0 6 0 .
On the right side, ∠ G F H = 2 1 ( 1 0 8 ° − 9 0 ° ) = 9 ° and ∠ F H G = 1 8 0 ° − ∠ G F H − ∠ F G H = 6 3 ° .
Then from the law of sines on △ F G H , sin 1 0 8 ° s 2 = sin 6 3 ° 1 , which solves to s 2 ≈ 1 . 0 6 7 .
Since s 2 > s 1 , the right square is larger.