$\frac{dy}{dx} = \frac{-x}{y}$

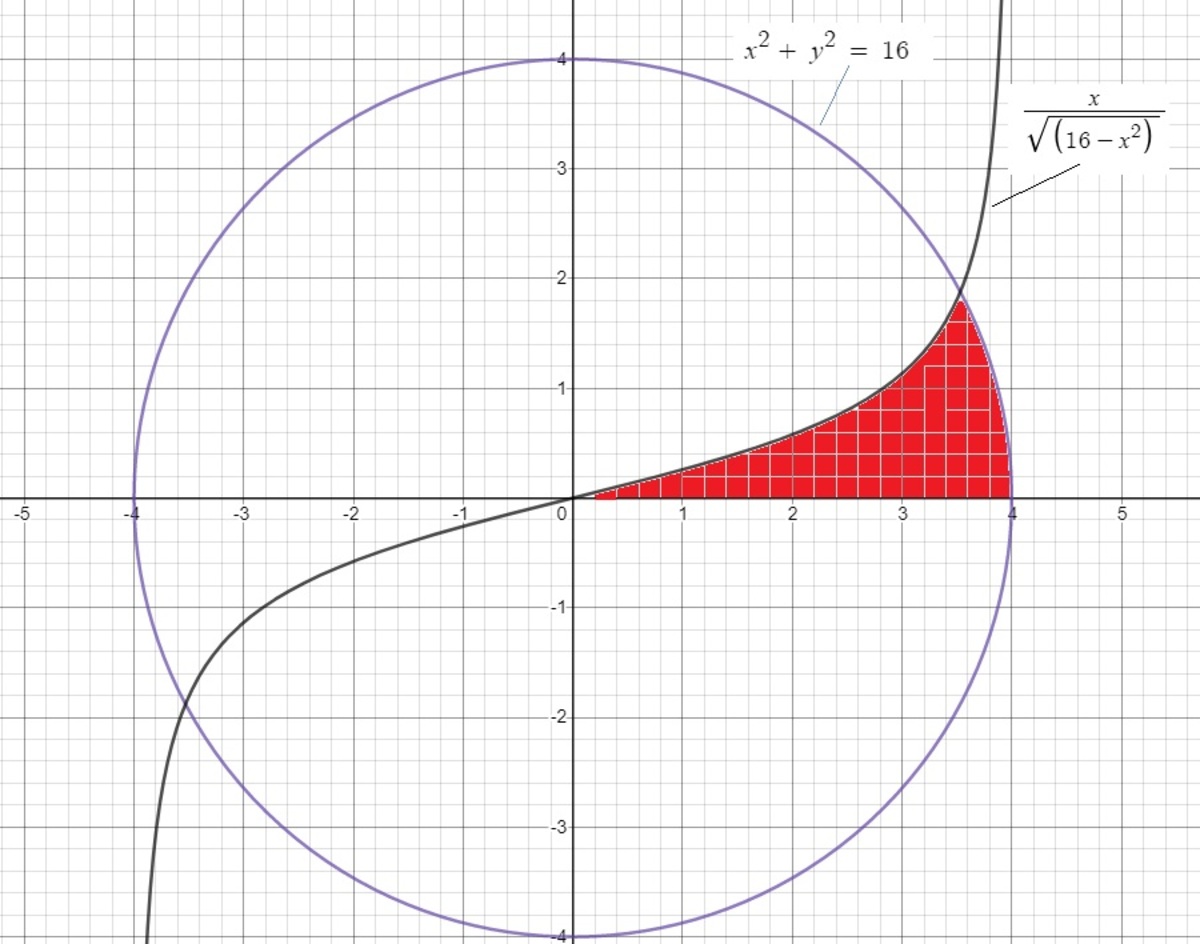
The above two curves intersect according to:

$\sqrt{16-x^2} = \frac{x}{\sqrt{16-x^2}} \Rightarrow x^2 + x - 16=0 \Rightarrow x = \frac{-1 \pm \sqrt{1 - 4(1)(-16)}}{2} = \frac{-1 \pm \sqrt{65}}{2}$

of which we only admit the positive root $x = \frac{\sqrt{65}-1}{2}.$ The red area is enclosed in the circle's first quadrant (which has area $\frac{1}{4} \cdot 16\pi = 4\pi).$ It can be directly computed per the integration:

$4\pi - \int_{0}^{(\sqrt{65}-1)/2} \sqrt{16-x^2} - \frac{x}{\sqrt{16-x^2}} dx \approx 2.7155$ (per Wolfram Alpha!)

and $\lfloor 1000 \cdot 2.7155 \rfloor = \boxed{2715}.$