Frictioned Plane
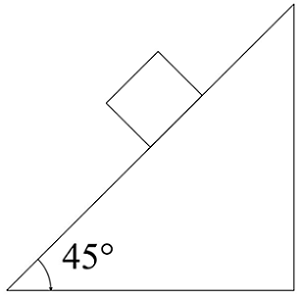
A block rests on a incline. What is the minimum coefficient of static friction necessary to keep the block in place?
Give your answer to 2 decimal places.
The answer is 1.00.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Start by summing the forces parallel to the incline.
Σ F ∥ = F f − m g sin ( 4 5 ∘ ) = 0 F f = m g sin ( 4 5 ∘ ) μ F N = m g sin ( 4 5 ∘ )
To find the normal force, sum the forces perpendicular to the incline.
Σ F ⊥ = F N − m g cos ( 4 5 ∘ ) = 0 F N = m g cos ( 4 5 ∘ )
Substitute this into our original equation.
μ m g cos ( 4 5 ∘ ) = m g sin ( 4 5 ∘ ) μ cos ( 4 5 ∘ ) = sin ( 4 5 ∘ ) μ = tan ( 4 5 ∘ ) = 1