Frisbee Bands
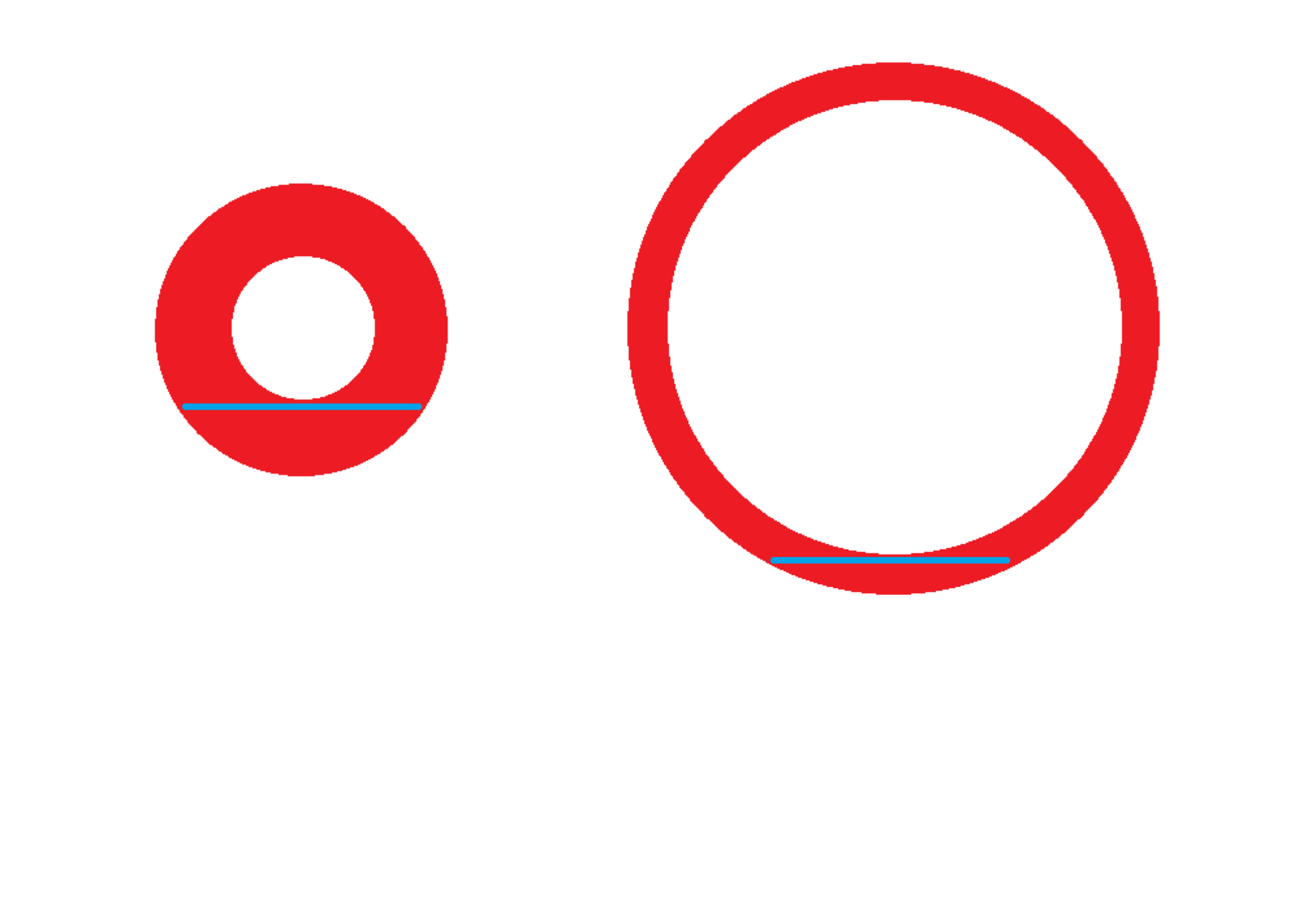
Two annuli are created such that each pair of outer and inner circles shares the same center though the right annulus has bigger outer radius, and the blue line is a tangent of the smaller circle within the bigger one as shown.
If the length of blue tangent is the same for both annuli, which one will have more area?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Since the blue line is the tangent, it can act as a base of right triangle with small radius r as the height, half blue length b as base, and bigger radius R as the hypotenuse. Thus, by Pythagorean theorem, R 2 = r 2 + b 2 .
And the annulus' area = π ( R 2 − r 2 ) = π ( b 2 ) .
Therefore, the annulus' area varies directly with the tangent's length: if the blue length is the same in both annuli, they both have the same area.