Gabriel's Horn
Gabriel's horn (also called Torricelli's trumpet), a geometric figure which has infinite surface area but finite volume, is formed by rotating the region bounded by the -axis, , and , around the -axis.
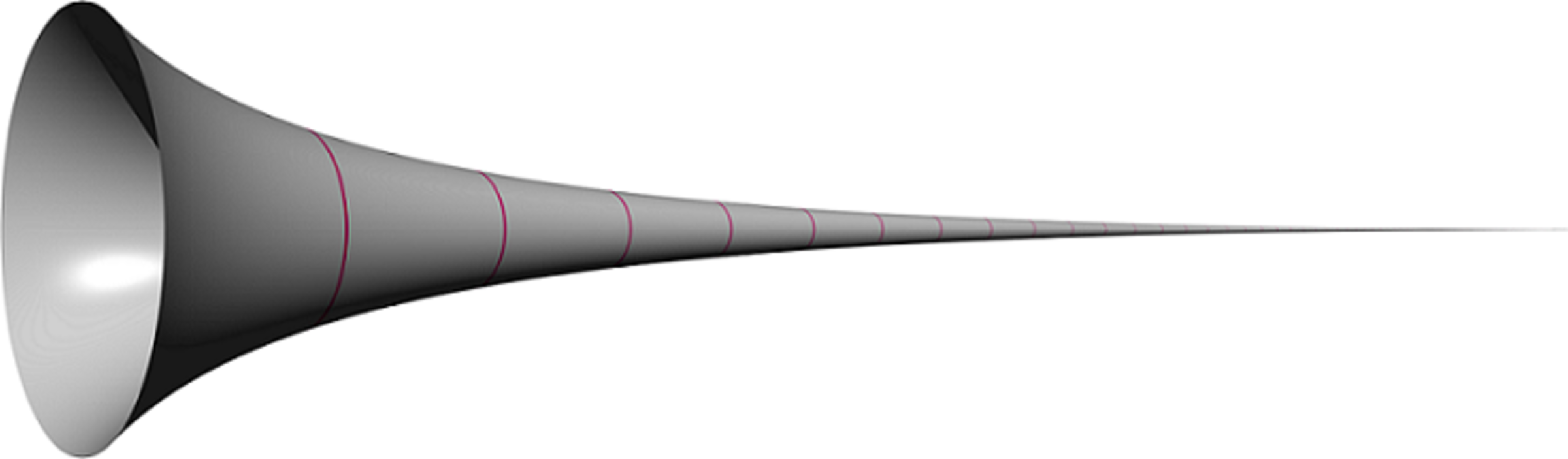
Find the volume of Gabriel's horn.
Bonus: Prove that Gabriel's horn has infinite surface area.
The answer is 3.14159.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
This simple shape can be cut up into vertical disks of radius y = x 1 and thickness d x whose volume is given by:
d V = π ( x 1 ) 2 d x = x 2 π d x
Integrating over the required range:
V = ∫ 1 ∞ x 2 π d x = π [ − x 1 ] 1 ∞ = − π ( ∞ 1 − 1 1 ) = π
So the volume is V = π
BONUS
We can once again use the vertical disks but this time focus on their differential surface area that makes up the surface of Gabriel's horn, that is:
d A = 2 π x 1 d x
Integrating gives:
A = 2 π ∫ 1 ∞ x 1 d x = 2 π [ ln x ] 1 ∞ = 2 π ( ln ( ∞ ) − ln ( 1 ) ) = ∞
So the surface area of Gabriel's horn is in fact infinite!