Square Inscribed Triangle

Let the side length of the triangle $AB=BC=CA = x$ and and that of the square $DE=EF=FG=GD=h$ .

We can see that $AG=GD=h$ and that:

$\begin{aligned} GC \sin{60^\circ} & = GF\\ (CA-AG)\sin{60^\circ} & = GF \\ (x-h)\sin{60^\circ} & = h \\ x\sin{60^\circ} & = \left( 1 +\sin{60^\circ} \right) h \end{aligned}$

We note that the area of $\triangle ABC$ is $\frac {1}{2}x^2\sin{60^\circ} = 1 \quad \Rightarrow x^2\sin{60^\circ} = 2$ .

$\begin{aligned} x\sin{60^\circ} & = \left( 1 +\sin{60^\circ} \right) h \\ x^2\sin^2{60^\circ} & = \left( 1 +\sin{60^\circ} \right)^2 h^2 \\ 2\sin{60^\circ} & = \left( 1 +2\sin{60^\circ} + \sin^2 {60^\circ} \right)h^2 \\ \sqrt{3} & = \left( 1 +\sqrt{3} + \dfrac {3}{4} \right)h^2 \\ 4 \sqrt{3} & = \left( 7 +4\sqrt{3} \right)h^2 \end{aligned}$

We note that the area of the square is:

$A=h^2 = \dfrac {4 \sqrt{3}}{7 +4\sqrt{3}} = \dfrac {(4 \sqrt{3})(7 - 4\sqrt{3})} {1} = 28\sqrt{3} - 48$

$\Rightarrow \dfrac{c-a}{b} = \dfrac {48-28}{3} = \dfrac {20}{3} = \boxed{6.667}$

First, we find the length of the side of the $\triangle$ . Since $AB = AC = BC$ :

$\dfrac{1}{2}(BC)^2\sin60^{\circ} = 1\\ (BC)^2\left(\dfrac{\sqrt{3}}{2}\right) = 2\\ (BC)^2 = \dfrac{4}{3^{\frac{1}{2}}}\\ BC = \dfrac{2}{3^{\frac{1}{4}}}$

Now, we let the side of the square be $q$ , as shown in the diagram below:

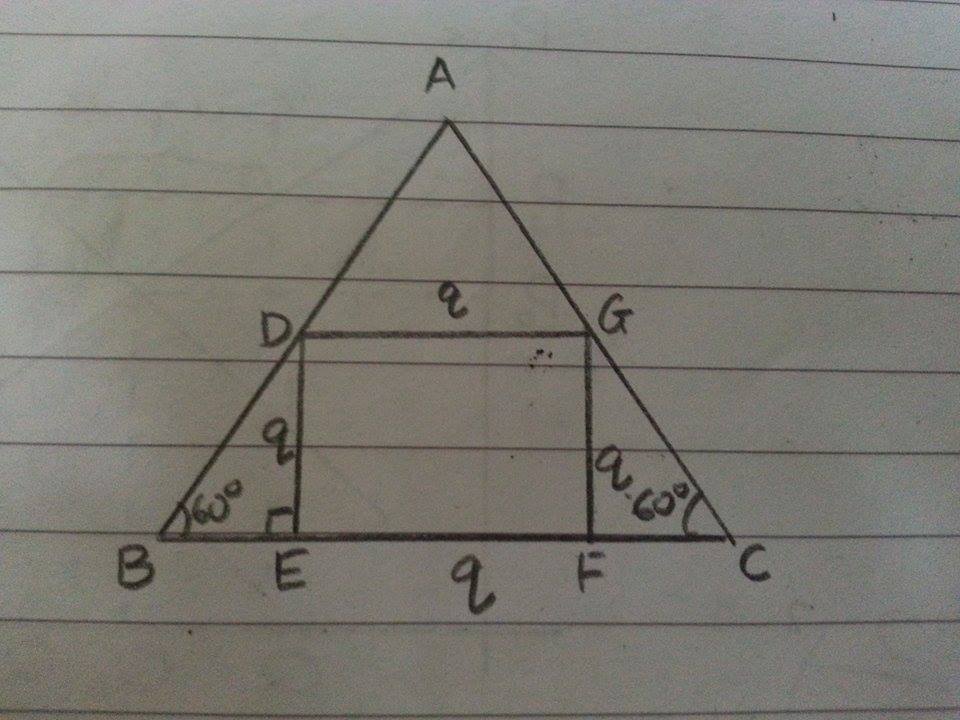
We can find $BE=FC$ in terms of $q$ :

$\tan60^{\circ} = \dfrac{DE}{BE}\\ \sqrt{3} = \dfrac{q}{BE}\\ BE = \dfrac{q}{\sqrt{3}}$

We know that:

$BC = BE + EF + FC\\ \dfrac{2}{3^{\frac{1}{4}}} = q + 2\dfrac{q}{\sqrt{3}}\\ \dfrac{2q+\sqrt{3}q}{\sqrt{3}} = \dfrac{2}{3^{\frac{1}{4}}}\\ q=\dfrac{2\sqrt{3}}{3^{\frac{1}{4}}\left(2+\sqrt{3}\right)}\\ q=\dfrac{2(3^{\frac{1}{4}})}{2 + \sqrt{3}}$

The area of the square is given as $q^2$ :

$q^2 = \left(\dfrac{2(3^{\frac{1}{4}})}{2 + \sqrt{3}}\right)^2\\ =\dfrac{4\sqrt{3}}{4+4\sqrt{3}+3}\\ =\dfrac{4\sqrt{3}}{7+4\sqrt{3}}\\ =\dfrac{4\sqrt{3}(7-4\sqrt{3})}{(7+4\sqrt{3})(7-4\sqrt{3})}\\ =\dfrac{28\sqrt{3} - 16(3)}{49-16(3)}\\ =\dfrac{28\sqrt{3}-48}{49-48}\\ =28\sqrt{3}-48$

$a\sqrt{b} - c=28\sqrt{3}-48\\ a=28,\;b=3,\;c=48\\ \dfrac{c-a}{b} = \dfrac{48-28}{3} = \dfrac{20}{3} = \boxed{6.667}$

Collection of shapes and spaces equate total the One

Call A, B are edges of square and equilateral triangles. $[ABC]=\frac{B^2\sqrt{3}}{4}=1 \Rightarrow B^2=\frac{4}{\sqrt{3}}$ $A\frac{2}{\sqrt{3}}+A=B$ $A=\frac{\sqrt{3}B}{\sqrt{3}+2}$ $A^2=\frac{3B^2}{7+4\sqrt{3}}=28\sqrt{3}-48$ $\frac{c-a}{b}=\frac{20}{3}=\boxed{6.667}$