Geometric shapes
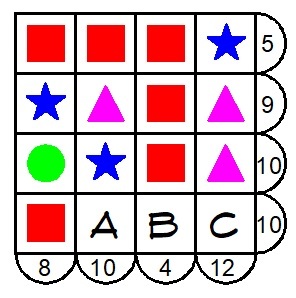In the diagram shown above, the value of each shape is positive integer. Find
.
Note:
The interpretation of the first row is: The sum of three squares and one star is five. The other rows and columns will follow.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
In column three, we can see that the sum is 4. Since there are already three squares, we can conclude that B is also a square so that each square has a value of one (note that the smallest positive integer is 1). So in row 1, the value of one star is 5-3=2. In row two, the value of two triangles is 9-2-1=6. So one triangle is 3. In row three, the value of one circle is 10-2-1-3=4. So A is 10-1-3-2=4 and C is 12-2-3-3=4.
The desired answer is A + B + C = 4 + 1 + 4 = 9