But I want to play Tic Tac Toe
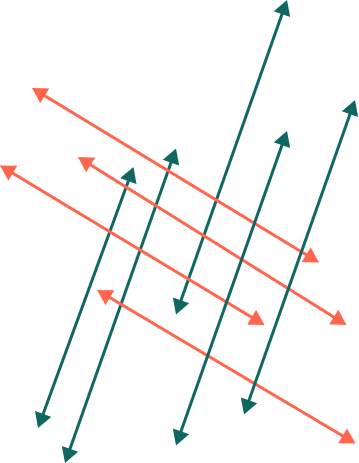
How many parallelograms are formed when a set of 5 parallel lines intersects a set of 4 parallel lines?
Details and Assumptions
- All parallel lines are extended indefinitely.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Discussions for this problem are now closed
I agree that image is, really, misleading...
i have a question sir .. how did you formulate the expression like n(n-1)/2 for the number of lines in respective directions ? i don't know the method to formulate it
just use the method of combination it will be much easier and same answer
Sir, I don't think the image is misleading as lines are rightly depicted with arrows..which by default indicates that that they are extend indefinitely(to the infinity).....had they been without arrows then they would have represented line segments which could have been misleading.
Equation for finding parallelograms when there are m lines intersecting n lines, can be calculated as m C 2 ⋅ n C 2 , where n C r represents n combination r, or n choose r. Or in another form, ( n r ) or ( n − r ) ! ⋅ r ! n ! . Subtitute m and n as 5 and 4, we get 60.
EDIT: The reason why m C 2 ⋅ n C 2 works is simple. There are m C 2 ways to choose two lines from m lines, and same for n lines. Multiply them, we get this equation.
This one is much more straightforward. There are 5C2 = 10 ways to choose two parallel lines from the set of five. There are 4C2 = 6 ways to choose two parallelograms from a set of four. Any parallelogram is uniquely determined by one pair of lines from the five, and one pair of lines from the four. Thus, the number of possible parallelograms is
(5C2) (4C2) = (10) (6) = 60
I dont understand this solution. What is C2 and how did you get 10 and 6
The image is misleading, all paralle lines are extended indefinitely. A parallelogram is formed from every set of two lines in one direction with every two lines in the other. There are n(n-1)/2 pairs of lines in one, and m(m-1)/2 pairs in the other direction (with n and m the number of lines). This means that there are nm(n-1)(m-1)/4 parallelograms. Here n = 5 and m = 4, yielding 60.