Geometry and Calculus of Binweed Flowers
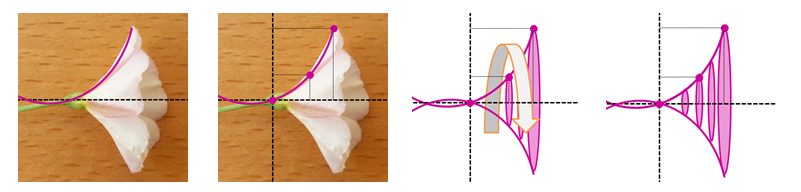
The flowers of binweeds have an interesting shape: a little bit like a cylinder with curved sides.
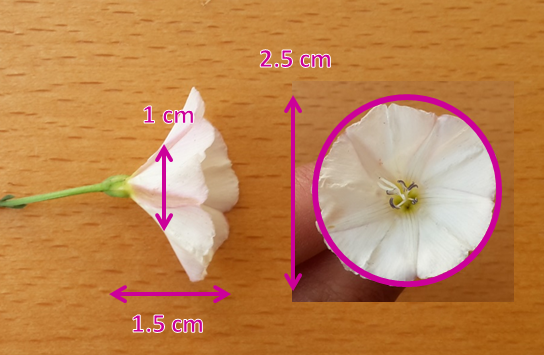
The flower is about perfectly symmetric, so if the height of the flower , the largest diameter on top and the diameter at half the height , which answer [ ] gets closes to the actual volume inside the calyx?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
There is a little calculus trick to get a quite accurate answer:
Splitting the flower in the middle, we recognize, that the curved side forms part of a square function.
If we draw three points (x-axis = height; y-axis = diameter): ( 0 ∣ 0 ) for the flower knot, which we set in the origin of the coordinate system, ( 0 . 7 5 ∣ 0 . 5 ) for the radius 2 1 ∗ 1 at half the height 2 1 ∗ 1 . 5 and ( 1 . 5 ∣ 1 . 2 5 ) for half the diameter at the top, we can arrange the following linear system (three times substitution of the square formula: y ( x ) = a ∗ x 2 + b ∗ x + c :
I.: 0 = a ∗ 0 2 + b ∗ 0 + c
II.: 0 . 5 = a ∗ 0 . 7 5 2 + 0 . 7 5 ∗ 0 + c
III.: 1 . 2 5 = a ∗ 1 . 5 2 + 1 . 5 ∗ 0 + c
And solving for a , b , c and substituting the general formula, we get y ( x ) = 0 . 2 2 2 ∗ x 2 + 0 . 5 ∗ x + 0 .
Imagine rotating this function around the x-axis. It will form the same body our flower encloses. To get this volume, we multiply the integral of the y ( x ) 2 with Π . That is a really cool calculus-formula! To understand it better: Imagine that calculating the square for every y ( x ) within the integral range with π equals calculating the area of a slice at x . And as an integral gives us the sum of the values, we mathematically "stack" those slices back on top of each other and get the volume.
Here in formulas:
π ∗ ∫ 1 . 5 0 ( 0 . 2 2 2 ∗ x 2 + 0 . 5 ∗ x ) 2 d x
π ∗ ∫ 1 . 5 0 ( 0 . 0 4 9 2 8 4 ∗ x 4 + 0 . 2 2 2 ∗ x 3 + 0 . 2 5 ∗ x 2 ) d x
and as we only have to calculate for the upper limit (as everything times and minus 0 stays the same) we get:
π ∗ ( 5 0 . 0 4 9 2 8 4 ∗ x 5 + 4 0 . 2 2 2 ∗ x 4 + 3 0 . 2 5 ∗ x 3 )
π ∗ ( 5 0 . 0 4 9 2 8 4 ∗ 1 . 5 5 + 4 0 . 2 2 2 ∗ 1 . 5 4 + 3 0 . 2 5 ∗ 1 . 5 3 )
= 2 . 0 0 1 c m 3
Here as visual process to follow along (without end-volume as I haven't found a fitting visualization):