Geometry Problem 101 Part 2
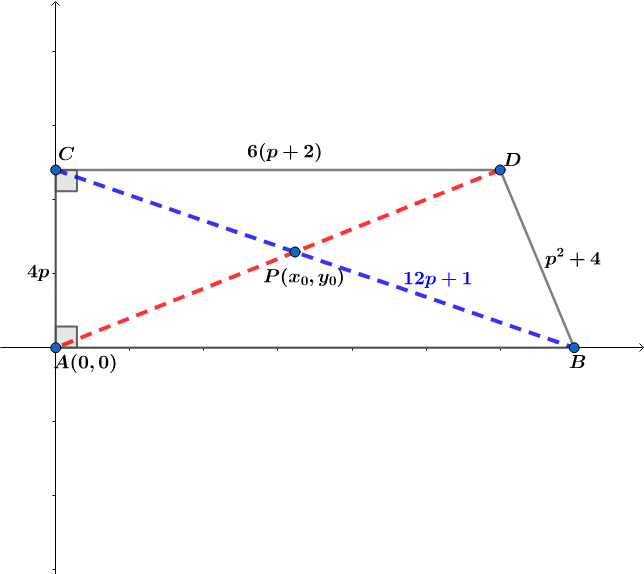
Let be a prime number and .
If diagonals and intersect at point and , where and are coprime positive integers, find .
The answer is 307.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let prime p > 2 .
For right △ D E B ( p 2 − 4 , 4 p , p 2 + 4 ) is a primitive pythagorean triple ⟹ A B = p 2 + 6 p + 8 .
For △ A B C ⟹ ( B C ) 2 = ( 1 2 p + 1 ) 2 = ( p 2 + 6 p + 8 ) 2 + ( 4 p ) 2 ⟹ p 4 + 1 2 p 3 − 7 6 p 2 + 7 2 p + 6 3 = 0 .
By inspection p = 3 is a root of p 4 + 1 2 p 3 − 7 6 p 2 + 7 2 p + 6 3 = 0 .
Performing long division we obtain: ( p − 3 ) ∗ ( p 3 + 1 5 p 2 − 3 1 p − 2 1 ) = 0 ⟹ p = 3 and prime p ≥ 3 ⟹ f ( p ) = p 3 + 1 5 p 2 − 3 1 p − 2 1 is monotonic increasing ⟹ f ( p ) ≥ f ( 3 ) = 4 8 ⟹ p = 3 is the only prime root satisfying p 4 + 1 2 p 3 − 7 6 p 2 + 7 2 p + 6 3 = 0 .
p = 3 ⟹ C : ( 0 , 1 2 ) , D : ( 3 0 , 1 2 ) and B : ( 3 5 , 0 ) ⟹ m B C = − 3 5 1 2 ⟹ y = − 3 5 1 2 ( x − 3 5 ) and m A D = 5 2 ⟹ y = 5 2 x
Solving the two equations above we obtain:
( x 0 = 1 3 2 1 0 , y 0 = 1 3 8 4 ) and x 0 + y 0 = 1 3 2 9 4 ⟹ m + n = 3 0 7 .
Note: ( p 2 − 4 , 4 p , p 2 + 4 ) is a primitive pythagorean triple for any prime p > 2 and ( 1 2 , 3 5 , 3 7 ) is also a primitive pythagorean triple.