Geometry with 1 and 2
Geometry
Level
3
A rectangle's two adjacent sides have lengths 1 and 2. The green points are the midpoints of two opposite sides.
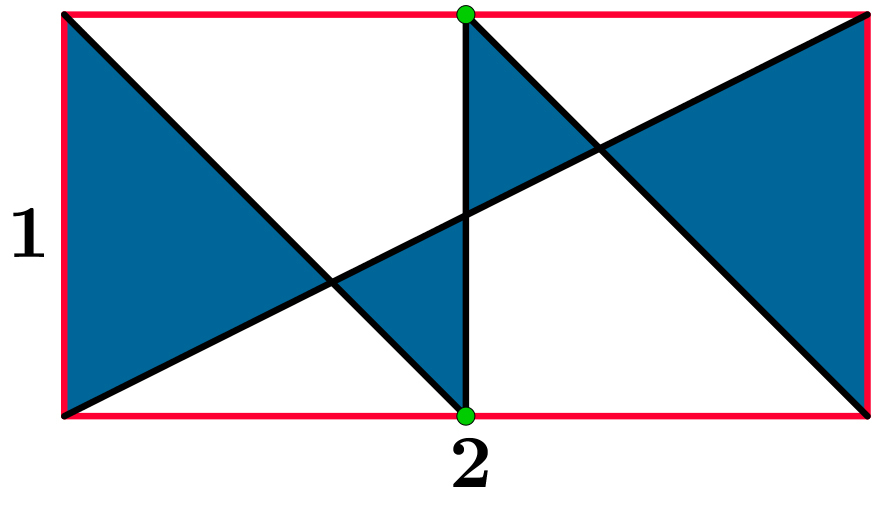
What is the sum of the blue areas?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
This is Sundar R 's solution:
. If we look at one half of the larger triangles , all we need are the heights of the blue triangles. Since the triangles are similar, the ratio of the altitudes is equal to the ratio of base lengths which is evidently 2:1. So the altitudes are 2/3 and 1/3. So the sum of the areas = =2 * (1/2 * 2/3 * 1 + 1/2 * 1/2 * 1/3) = 2(1/3 + 1/12) = 2(5/12) = 5/6