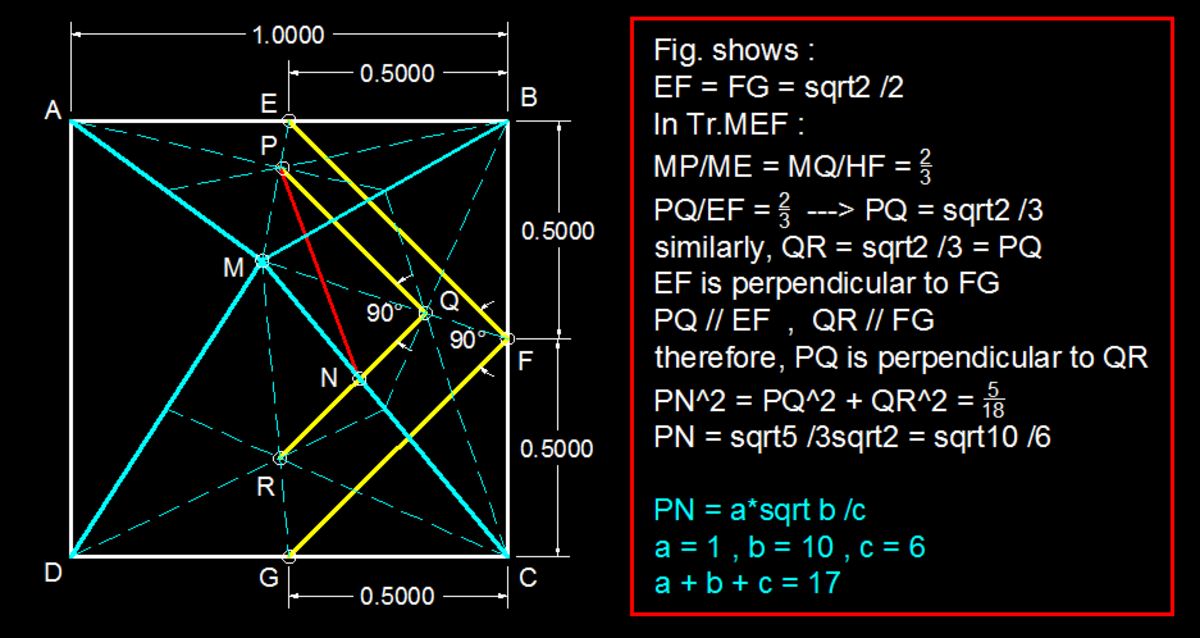
Geometry with a Square

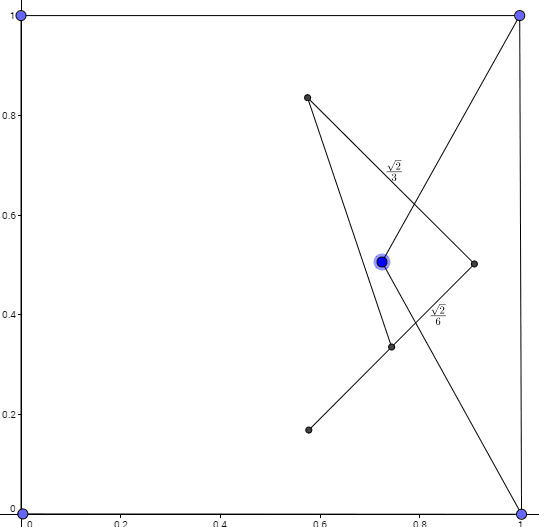
Images from https://app.geogebra.org/#geometry

Since P,Q and R are the intersections of medians, they are the centroids of $\triangle ABM$ , $\triangle BCM$ and $\triangle CDM$ .

For clearer illustration, I have drawn 2 squares, square X on the left and square Y on the right.

A property of centroids is that the distance from a vertex to the centroid is $\frac {2}{3}$ the length of the median.

Let J be the midpoint of MB and K be the midpoint of MC.

In square X, $\triangle PJQ \sim \triangle AJC$ so $PQ \| AC$ and $PQ = \frac {1}{3} AC = \frac {\sqrt{2}} {3}$

Similarly, in square Y, $\triangle QKR \sim \triangle BKD$ so $QR \| BD$ and $QR = \frac {1}{3} BD = \frac {\sqrt{2}} {3}$

Also, AC and BD are perpendicular. Since $PQ \| AC$ and $QR \| BD$ , we see that $\angle PQR = 90 ^ \circ$ .

Direct application of Pythagorean Theorem gives $Distance = \sqrt {(\frac {\sqrt {2}}{6})^2 + (\frac {\sqrt {2}}{3})^2} = \frac {1*\sqrt {10}}{6}$

$\Rightarrow a+b+c = 1+10+6 =\boxed {17}$