Go The Distance

Geometry Level 3

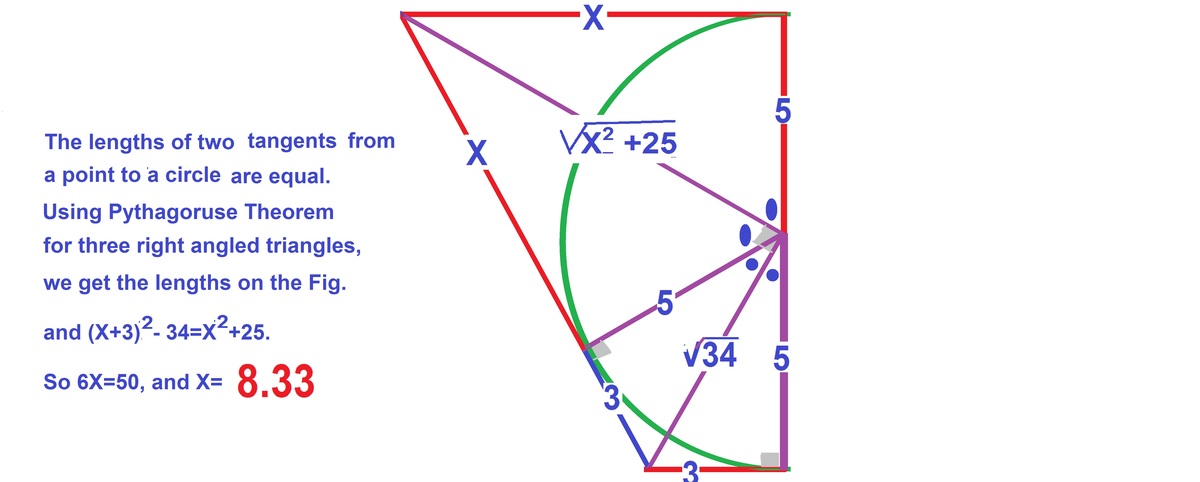
A semicircle is drawn on the side with length 10 of a rectangle, as shown in the diagram above.

Then a line is drawn from the point 3 units to the left of the bottom right corner, such that it is tangent to the semicircle.

Where do this line and the upper side of the rectangle intersect?

Enter the distance $X$ , correct to 2 decimal places.

The answer is 8.33.

2 solutions

Yee-Lynn Lee
Aug 29, 2016

The line drawn starting from the point 3 units to the left of the bottom corner and tangent to the semi-circle has a length of $X+3$ by the Two Tangents Theorem (Ice Cream Cone Theorem, Hat Theorem, etc.), which states that given a circle, if P is any point lying outside the circle, and if A and B are points such that PA and PB are tangent to the circle, then PA = PB.

By the Pythagorean Theorem , $(X-3)^2+10^2=(X+3)^2$ . Solving for $X$ , we get $X=8.33$ .

Elegant solution. :) (It took me a minute to realize that you applied the TTT twice to establish the $X + 3$ length.)

Brian Charlesworth - 4 years, 9 months ago

Thanks! :)

Yee-Lynn Lee - 4 years, 9 months ago

Did the same .

vishwash kumar - 4 years, 8 months ago

Niranjan Khanderia - 4 years, 8 months ago

Ujjwal Rane
Sep 2, 2016

Tangent to Semicircle in Rectangle

The angles made by the tangent with the top and bottom edge of the rectangle are supplementary. So half of these angles $\alpha$ and $\beta$ will be complementary.

So $\tan \alpha = \frac{5}{x} = \cot \beta = \frac{3}{5}$ giving $x = \frac{25}{3} = 8.3333$

Beautiful short solution. +1)

Niranjan Khanderia - 4 years, 8 months ago

Thank you sir!

Ujjwal Rane - 4 years, 8 months ago

Go The Distance

The answer is 8.33.

2 solutions

0 pending reports