A geometry problem by A Former Brilliant Member

Geometry Level 4

The sides of a triangle are in an arithmetic progression , and the greatest angle exceeds the least angle by 90 degrees.

If the sides of this triangle are in the ratio of $\sqrt{m} + 1 : \sqrt m : \sqrt m - 1$ , find the value of $m^2-45$ .

The answer is 4.

2 solutions

Ayush G Rai
Oct 22, 2016

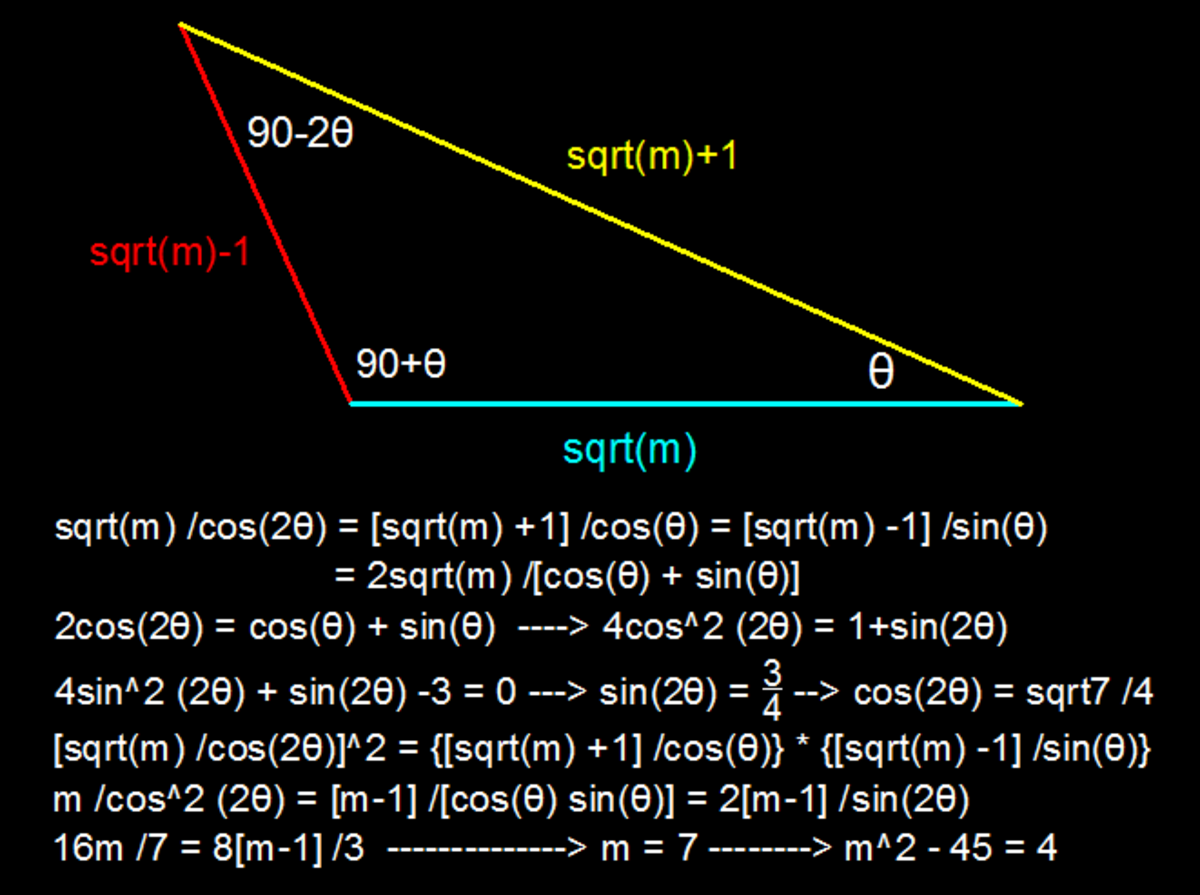
If $a,b,c$ where $a>b>c⟹a+c=2b$
$\dfrac{a}{sin(90+x)}=\dfrac{b}{sin(90-2x)}=\dfrac{c}{sinx}=2R$
$⟹a=2Rcosx,b=2Rcos2x,c=2Rsinx$
Using $a+c=2b,cosx+sinx=2cos2x=2(cosx-sinx)(cosx+sinx)$
As $(90-2x)>0,cos2x>0$
and also $cosx,sinx>0⟹cosx+sinx>0$
Cancelling $cosx+sinx,$ we get $cosx-sinx=\dfrac{1}{2}$
Squaring we get, $1-sin2x=\dfrac{1}{4}⟺sin2x=?$
$⟹cos2x=+\sqrt{1-sin^22x}=\dfrac{\sqrt7}{4}$
$cos^2x=\dfrac{1+cos2x}{2}=\dfrac{4+\sqrt7}{8}={(\dfrac{\sqrt7+1}{4})}^2$
Find $sin^2x$ and use $cosx,sinx>0.$
After this we get our required ratios as $\boxed{\sqrt7+1:\sqrt7:\sqrt7-1}.$

I used sin rule only, just solved the equation without considering 2R, that too yields the result. But unfortunately I submitted -38 and clicked discuss solution, then saw it was m^2-45!

Prince Loomba - 4 years, 7 months ago

haha lol.bad luck loomba.better luck next time

Ayush G Rai - 4 years, 7 months ago

Ahmad Saad
Nov 2, 2016

A geometry problem by A Former Brilliant Member

The answer is 4.

2 solutions

0 pending reports